The Rational Zero Theorem and Solving Polynomial Equations
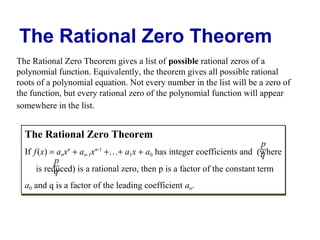
- 1. The Rational Zero Theorem The Rational Zero Theorem gives a list of possible rational zeros of a polynomial function. Equivalently, the theorem gives all possible rational roots of a polynomial equation. Not every number in the list will be a zero of the function, but every rational zero of the polynomial function will appear somewhere in the list. The Rational Zero Theorem p If f (x) = anx + an-1x +…+ a1x + a0 has integer coefficients and (where q n n-1 1 0 p is reduced) is a rational zero, then p is a factor of the constant term q n n n-1 n-1 a0 and q is a factor of the leading coefficient an. 0 n
- 2. EXAMPLE: Using the Rational Zero Theorem List all possible rational zeros of f (x) = 15x3 + 14x2 − 3x – 2. Solution The constant term is –2 and the leading coefficient is 15. Factors of the constant term, − 2 Factors of the leading coefficient, 15 ±1, ± 2 = ±1, ± 3, ± 5, ± 15 Possible rational zeros = = ±1, ± 2, 2 ± 1, ± 3, 3 2 ± 1, ± 5, 5 1 2 ± 15 , ± 15 Divide ±1 and ± 2 by ± 1. Divide ±1 and ± 2 by ± 3. Divide ±1 and ± 2 by ± 5. Divide ±1 and ± 2 by ± 15. There are 16 possible rational zeros. The actual solution set to f (x) = 15x3 + 14x2 − 3x – 2 = 0 is {-1, −1/3, 2/5}, which contains 3 of the 16 possible solutions.
- 3. EXAMPLE: Solve: Solving a Polynomial Equation x4 − 6x2 − 8x + 24 = 0. Solution Because we are given an equation, we will use the word "roots," rather than "zeros," in the solution process. We begin by listing all possible rational roots. Factors of the constant term, 24 Factors of the leading coefficient, 1 ±1, ± 2 ± 3, ± 4, ± 6, ± 8, ± 12, ± 24 = ±1 = ±1, ± 2 ± 3, ± 4, ± 6, ± 8, ± 12, ± 24 Possible rational zeros =
- 4. EXAMPLE: Solve: Solving a Polynomial Equation x4 − 6x2 − 8x + 24 = 0. Solution The graph of f (x) = x4 − 6x2 − 8x + 24 is shown the figure below. Because the x-intercept is 2, we will test 2 by synthetic division and show that it is a root of the given equation. 2 1 1 0 −6 −8 24 2 4 −4 −24 2 −2 −12 0 The zero remainder indicates that 2 is a root of x4 − 6x2 − 8x + 24 = 0. x-intercept: 2
- 5. EXAMPLE: Solve: Solving a Polynomial Equation x4 − 6x2 − 8x + 24 = 0. Solution Now we can rewrite the given equation in factored form. x4 − 6x2 + 8x + 24 = 0 (x – 2)(x3 + 2x2 − 2x − 12) = 0 x–2=0 or x3 + 2x2 − 2x − 12 = 0 This is the given equation. This is the result obtained from the synthetic division. Set each factor equal to zero. Now we must continue by factoring x3 + 2x2 - 2x - 12 = 0
- 6. EXAMPLE: Solve: Solving a Polynomial Equation x4 − 6x2 − 8x + 24 = 0. Solution Because the graph turns around at 2, this means that 2 is a root of even multiplicity. Thus, 2 must also be a root of x3 + 2x2 − 2x − 12 = 0. These are the coefficients of x3 + 2x2 − 2x − 12 = 0. 2 1 1 x-intercept: 2 2 2 4 −2 −12 8 12 6 0 The zero remainder indicates that 2 is a root of x3 + 2x2 − 2x − 12 = 0.
- 7. EXAMPLE: Solve: Solution Solving a Polynomial Equation x4 − 6x2 − 8x + 24 = 0. Now we can solve the original equation as follows. x4 − 6x2 + 8x + 24 = 0 This is the given equation. (x – 2)(x3 + 2x2 − 2x − 12) = 0 (x – 2)(x – 2)(x2 + 4x + 6) = 0 x–2=0 x=2 or This was obtained from the first synthetic division. This was obtained from the second synthetic division. x–2=0 x=2 or x2 + 4x + 6 = 0 Set each factor equal to zero. x2 + 4x + 6 = 0 Solve.
- 8. EXAMPLE: Solve: Solution Solving a Polynomial Equation x4 − 6x2 − 8x + 24 = 0. We can use the quadratic formula to solve x2 + 4x + 6 = 0. −b ± b 2 − 4ac x= 2a −4 ± 42 − 4 ( 1) ( 6 ) = 2 ( 1) − 4 ± −8 = 2 −4 ± 2i 2 = 2 = −2 ± i 2 We use the quadratic formula because x2 + 4x + 6 = 0 cannot be factored. Let a = 1, b = 4, and c = 6. Multiply and subtract under the radical. −8 = 4(2)(−1) = 2i 2 Simplify. The solution set of the original equation is {2, −2 − i 2, −2 + ii 2 }.
- 9. Properties of Polynomial Equations 1. If a polynomial equation is of degree n, then counting multiple roots separately, the equation has n roots. 2. If a + bi is a root of a polynomial equation (b ≠ 0), then the non-real complex number a − bi is also a root. Non-real complex roots, if they exist, occur in conjugate pairs.
- 10. Descartes' Rule of Signs n n−1 2 … If f (x) = anxn + an−1xn−1 + … + a2x2 + a1x + a0 be a polynomial with real n n−1 2 1 0 coefficients. 1. The number of positive real zeros of f is either equal to the number of sign changes of f (x) or is less than that number by an even integer. If there is only one variation in sign, there is exactly one positive real zero. 2. The number of negative real zeros of f is either equal to the number of sign changes of f (−x) or is less than that number by an even integer. If f (−x) has only one variation in sign, then f has exactly one negative real zero.
- 11. EXAMPLE: Using Descartes’ Rule of Signs Determine the possible number of positive and negative real zeros of f (x) = x3 + 2x2 + 5x + 4. Solution 1. To find possibilities for positive real zeros, count the number of sign changes in the equation for f (x). Because all the terms are positive, there are no variations in sign. Thus, there are no positive real zeros. 2. To find possibilities for negative real zeros, count the number of sign changes in the equation for f (−x). We obtain this equation by replacing x with −x in the given function. f (x) = x3 + 2x2 + 5x + 4 Replace x with −x. f (−x) = (−x)3 + 2(−x)2 + 5(−x) + 4 = −x3 + 2x2 − 5x + 4 This is the given polynomial function.
- 12. EXAMPLE: Using Descartes’ Rule of Signs Determine the possible number of positive and negative real zeros of f (x) = x3 + 2x2 + 5x + 4. Solution Now count the sign changes. f (−x) = −x3 + 2x2 − 5x + 4 1 2 3 There are three variations in sign. # of negative real zeros of f is either equal to 3, or is less than this number by an even integer. This means that there are either 3 negative real zeros or 3 − 2 = 1 negative real zero.