➥🔝 7737669865 🔝▻ jhansi Call-girls in Women Seeking Men 🔝jhansi🔝 Escorts S...
Columns lecture#4
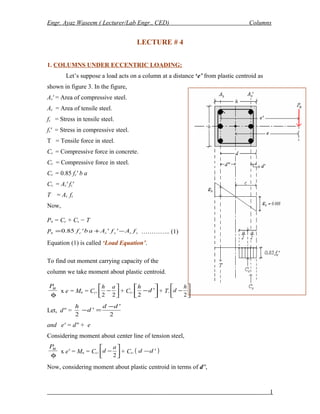
1. Engr. Ayaz Waseem ( Lecturer/Lab Engr., CED) Columns
LECTURE # 4
1. COLUMNS UNDER ECCENTRIC LOADING:
Let’s suppose a load acts on a column at a distance ‘e’ from plastic centroid as
shown in figure 3. In the figure,
As′ = Area of compressive steel.
As = Area of tensile steel.
fs = Stress in tensile steel.
fs′ = Stress in compressive steel.
T = Tensile force in steel.
Cc = Compressive force in concrete.
Cs = Compressive force in steel.
Cc = 0.85 fc′ b a
Cs = As′ fs′
T = As fs
Now,
Pn = Cc + Cs − T
Pn ssssc fAfAabf −+= '''85.0 ………….. (1)
Equation (1) is called ‘Load Equation’.
To find out moment carrying capacity of the
column we take moment about plastic centroid.
φ
uP
x e = Mn = Cc.
−
22
ah
+ Cs.
− '
2
d
h
+ T.
−
2
h
d
Let, d′′ =
2
'
'
2
dd
d
h −
=−
and e′ = d′′ + e
Considering moment about center line of tension steel,
φ
uP
x e′ = Mn = Cc.
−
2
a
d + Cs.( )'dd −
Now, considering moment about plastic centroid in terms of d′′,
1
2. Engr. Ayaz Waseem ( Lecturer/Lab Engr., CED) Columns
Pn x e = Mn = Cc. + Cs.[d − d′′ − d′]+ T. ( )d ′′
Mn = 0.85 fc’ b a. + As’ fs’.[d − d′′ − d′]+ As fs. ( )d ′′ ……………….. (2)
Equation (2) is called ‘Moment Equation’.
Case 1: PURE AXIAL LOAD/CRUSHING FAILURE:
We know that,
Pn = Cc + Cs − T
But in this case there is pure compression,
so, no tension steel is present
Ast = As + As′ and Cs = C1 + C2
Pn ystcc fAAf. +′850=
Pn yststgc fAAAf. +)(′850=
Pn = 0.85 fc′ Ag + ( fy – 0.85 fc′ ) Ast
Mn = 0
Case 2: BALANCE FAILURE:
For balanced condition,
a = ab = β1 cb ……………………..…………. (1)
Fitst we need to find cb, in figure comparing ∆ c f g and
∆ a b c we get,
If we want to express cb in terms of fy then,
2
LOAD EQUATION
MOMENT EQUATION
3. Engr. Ayaz Waseem ( Lecturer/Lab Engr., CED) Columns
Using Es = 200, 000 MPa and rearranging,
y
b
f
d
c
+600
600
= ……………………………..... (3)
Using equation (2) in equation (1) we get,
=ba β1
Now, in figure comparing ∆ c f g and ∆ c d e, we get,
If εs′ < εy , then fs′ = Es. εs′
If εs′ ≥ εy , then fs′ = fy
and for balanced failure we already know that fs = fy
So,
Pn ysssbc fAfAabf −+= '''85.0
If As = As′ and fs′ = fy , then
Pn = 0.85 fc′ b ba
and
[ ] ( )dfAdddfA
a
ddabfM ysss
b
bcn ′′+′−′′−′′+
−′′−′= .
2
..85.0
3
LOAD EQUATIONS
MOMENT
EQUATION
4. Engr. Ayaz Waseem ( Lecturer/Lab Engr., CED) Columns
Case 3: PURE FLEXURAL FAILURE:
In this case no axial load is acting is
acting on the column and column just
behaves like a beam. Therefore,
Pn = 0
In figure comparing ∆ a b c and ∆ c f g, we
get,
cdc
s
−
=
ε003.0
ccd s ×=− ε003.0003.0
s
d
c
ε+
=
003.0
003.0
………………………. (1)
Note: In equation (1) value of ‘c’ can not be found out because even though we know
that εs >> ε y but we don’t know the exact value of εs . Whereas in case of balance failure
‘c’ could be found out as the equation involved ε y rather than εs .
Now, in figure comparing ∆ a b c and ∆ c d e, we get,
dcc
s
′−
′
=
ε003.0
1
1
003.0
β
β
ε ×
′−
=′
c
dc
s
′−
=′
c
dc
s
1
11
003.0
β
ββ
ε
′−
=′
a
da
s
1
003.0
β
ε ……………… (2)
Now,
sssscn fAfAabfP −′′+′= ..85.0
For pure flexural failure we know that, fs = fy and Pn = 0, so
4
LOAD EQUATION
5. Engr. Ayaz Waseem ( Lecturer/Lab Engr., CED) Columns
ysssc fAfAabf −′′+′= ..85.00 …………. (3)
Assuming, fs′ = fy and As′ = As , we get
0..85.00 =⇒′= aabfc
This means that concrete is not taking any load which is not possible. So, our assumption
is wrong. Therefore, compression steel can not yield at pure flexural failure point.
Now, as εs′ < εy , so
fs′ = Es. εs′
Using equation (2) in above equation, we get,
′−
×=′
a
da
fs
1
003.0000,200
β
′−
=′
a
da
fs
1
600
β
………………….. (4)
Now, using equation (4) in (3), we get,
yssc fA
a
da
Aabf −
′−
×′+′= 1
600..85.00
β
………………….. (5)
Equation (5) results in a quadratic equation. We solve above equation for the value of ‘a’
and then using equation (4) we find fs′.
[ ] ( )dfAdddfA
a
ddabfM yssscn ′′+′−′′−′′+
−′′−′= .
2
..85.0
Now, using the values of a and fs′ in moment equation we can easily find value of Mn ,
whereas, value of Pn is already known i.e., Pn = 0.
5
Es = 200,000 MPa
MOMENT
EQUATION
6. Engr. Ayaz Waseem ( Lecturer/Lab Engr., CED) Columns
ysssc fAfAabf −′′+′= ..85.00 …………. (3)
Assuming, fs′ = fy and As′ = As , we get
0..85.00 =⇒′= aabfc
This means that concrete is not taking any load which is not possible. So, our assumption
is wrong. Therefore, compression steel can not yield at pure flexural failure point.
Now, as εs′ < εy , so
fs′ = Es. εs′
Using equation (2) in above equation, we get,
′−
×=′
a
da
fs
1
003.0000,200
β
′−
=′
a
da
fs
1
600
β
………………….. (4)
Now, using equation (4) in (3), we get,
yssc fA
a
da
Aabf −
′−
×′+′= 1
600..85.00
β
………………….. (5)
Equation (5) results in a quadratic equation. We solve above equation for the value of ‘a’
and then using equation (4) we find fs′.
[ ] ( )dfAdddfA
a
ddabfM yssscn ′′+′−′′−′′+
−′′−′= .
2
..85.0
Now, using the values of a and fs′ in moment equation we can easily find value of Mn ,
whereas, value of Pn is already known i.e., Pn = 0.
5
Es = 200,000 MPa
MOMENT
EQUATION
![Engr. Ayaz Waseem ( Lecturer/Lab Engr., CED) Columns
Pn x e = Mn = Cc. + Cs.[d − d′′ − d′]+ T. ( )d ′′
Mn = 0.85 fc’ b a. + As’ fs’.[d − d′′ − d′]+ As fs. ( )d ′′ ……………….. (2)
Equation (2) is called ‘Moment Equation’.
Case 1: PURE AXIAL LOAD/CRUSHING FAILURE:
We know that,
Pn = Cc + Cs − T
But in this case there is pure compression,
so, no tension steel is present
Ast = As + As′ and Cs = C1 + C2
Pn ystcc fAAf. +′850=
Pn yststgc fAAAf. +)(′850=
Pn = 0.85 fc′ Ag + ( fy – 0.85 fc′ ) Ast
Mn = 0
Case 2: BALANCE FAILURE:
For balanced condition,
a = ab = β1 cb ……………………..…………. (1)
Fitst we need to find cb, in figure comparing ∆ c f g and
∆ a b c we get,
If we want to express cb in terms of fy then,
2
LOAD EQUATION
MOMENT EQUATION](data:image/gif;base64,R0lGODlhAQABAIAAAAAAAP///yH5BAEAAAAALAAAAAABAAEAAAIBRAA7)