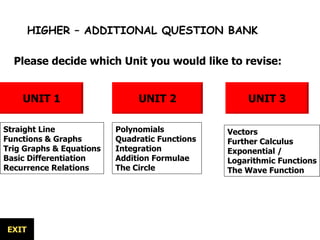
Higher revision 1, 2 & 3
- 1. HIGHER – ADDITIONAL QUESTION BANK EXIT UNIT 1 UNIT 2 UNIT 3 Please decide which Unit you would like to revise: Straight Line Functions & Graphs Trig Graphs & Equations Basic Differentiation Recurrence Relations Polynomials Quadratic Functions Integration Addition Formulae The Circle Vectors Further Calculus Exponential / Logarithmic Functions The Wave Function
- 2. HIGHER – ADDITIONAL QUESTION BANK UNIT 1 : Functions & Graphs Straight Line Recurrence Relations Basic Differentiation Trig Graphs & Equations EXIT
- 3. HIGHER – ADDITIONAL QUESTION BANK UNIT 1 : Straight Line You have chosen to study: Please choose a question to attempt from the following: 1 2 3 4 5 EXIT Back to Unit 1 Menu
- 4. STRAIGHT LINE : Question 1 Find the equation of the straight line which is perpendicular to the line with equation 3x – 5y = 4 and which passes through the point (-6,4). Go to full solution Go to Marker’s Comments Go to Straight Line Menu Go to Main Menu Reveal answer only EXIT
- 5. STRAIGHT LINE : Question 1 Find the equation of the straight line which is perpendicular to the line with equation 3x – 5y = 4 and which passes through the point (-6,4). Go to full solution Go to Marker’s Comments Go to Straight Line Menu Go to Main Menu Reveal answer only EXIT y = -5 / 3 x - 6
- 6. Markers Comments Begin Solution Continue Solution 3x – 5y = 4 3x - 4 = 5y 5y = 3x - 4 ( 5) y = 3 / 5 x - 4 / 5 Using y = mx + c , gradient of line is 3 / 5 So required gradient = -5 / 3 , ( m 1 m 2 = -1) We now have (a,b) = (-6,4) & m = -5 / 3 . Using y – b = m(x – a) We get y – 4 = -5 / 3 (x – (-6)) y – 4 = -5 / 3 (x + 6) y – 4 = -5 / 3 x - 10 Question 1 Find the equation of the straight line which is perpendicular to the line with equation 3x – 5y = 4 and which passes through the point (-6,4). Straight Line Menu y = -5 / 3 x - 6 Back to Home
- 8. Go to full solution Go to Marker’s Comments Go to Straight Line Menu Go to Main Menu Reveal answer only EXIT STRAIGHT LINE : Question 2 Find the equation of the straight line which is parallel to the line with equation 8x + 4y – 7 = 0 and which passes through the point (5,-3).
- 9. Go to full solution Go to Marker’s Comments Go to Straight Line Menu Go to Main Menu Reveal answer only EXIT STRAIGHT LINE : Question 2 Find the equation of the straight line which is parallel to the line with equation 8x + 4y – 7 = 0 and which passes through the point (5,-3). y = -2x + 7
- 10. Markers Comments Begin Solution Continue Solution Question 2 Straight Line Menu 8x + 4y – 7 = 0 4y = -8x + 7 ( 4) y = -2x + 7 / 4 y = -2x + 7 Using y = mx + c , gradient of line is -2 So required gradient = -2 as parallel lines have equal gradients. We now have (a,b) = (5,-3) & m = -2 . Using y – b = m(x – a) We get y – (-3) = -2(x – 5) y + 3 = -2x + 10 Find the equation of the straight line which is parallel to the line with equation 8x + 4y – 7 = 0 and which passes through the point (5,-3). Back to Home
- 12. Go to full solution Go to Marker’s Comments Go to Straight Line Menu Go to Main Menu Reveal answer only EXIT STRAIGHT LINE : Question 3 In triangle ABC, A is (2,0), B is (8,0) and C is (7,3). (a) Find the gradients of AC and BC. (b) Hence find the size of ACB. X Y A B C
- 13. Go to full solution Go to Marker’s Comments Go to Straight Line Menu Go to Main Menu Reveal answer only EXIT STRAIGHT LINE : Question 3 In triangle ABC, A is (2,0), B is (8,0) and C is (7,3). (a) Find the gradients of AC and BC. (b) Hence find the size of ACB. = 77.4 ° (b) m AC = 3 / 5 m BC = - 3 (a) X Y A B C
- 16. STRAIGHT LINE : Question 4 Go to full solution Go to Marker’s Comments Go to Straight Line Menu Go to Main Menu Reveal answer only EXIT In triangle PQR the vertices are P(4,-5), Q(2,3) and R(10-1). X Y P(4,-5) Q(2,3) R(10,-1) Find (a) the equation of the line e, the median from R of triangle PQR. (b) the equation of the line f, the perpendicular bisector of QR. (c) The coordinates of the point of intersection of lines e & f.
- 17. STRAIGHT LINE : Question 4 Go to full solution Go to Marker’s Comments Go to Straight Line Menu Go to Main Menu Reveal answer only EXIT In triangle PQR the vertices are P(4,-5), Q(2,3) and R(10-1). X Y P(4,-5) Q(2,3) R(10,-1) Find (a) the equation of the line e, the median from R of triangle PQR. (b) the equation of the line f, the perpendicular bisector of QR. (c) The coordinates of the point of intersection of lines e & f. y = -1 (a) y = 2x – 11 (b) (5,-1) (c)
- 19. Markers Comments Begin Solution Continue Solution Question 4 (b) Straight Line Menu (b) the equation of the line f, the perpendicular bisector of QR. In triangle PQR the vertices are P(4,-5), Q(2,3) and R(10-1). Find = - 1 / 2 required gradient = 2 (m 1 m 2 = -1) Using y – b = m(x – a) with (a,b) = (6,1) & m = 2 we get y – 1 = 2(x – 6) Solution to 4 (c) Back to Home X Y P(4,-5) Q(2,3) R(10,-1) (b) Midpoint of QR is (6,1) m QR = 3 – (-1) 2 - 10 = 4 / -8 so f is y = 2x – 11
- 20. Markers Comments Begin Solution Continue Solution Question 4 (c) Straight Line Menu In triangle PQR the vertices are P(4,-5), Q(2,3) and R(10-1). Find (c) The coordinates of the point of intersection of lines e & f. (c) e & f meet when y = -1 & y = 2x -11 so 2x – 11 = -1 ie 2x = 10 ie x = 5 Point of intersection is (5,-1) Back to Home X Y P(4,-5) Q(2,3) R(10,-1)
- 24. STRAIGHT LINE : Question 5 Go to full solution Go to Marker’s Comments Go to Straight Line Menu Go to Main Menu Reveal answer only EXIT In triangle EFG the vertices are E(6,-3), F(12,-5) and G(2,-5). Find (a) the equation of the altitude from vertex E. (b) the equation of the median from vertex F. (c) The point of intersection of the altitude and median. X Y G(2,-5) E(6,-3) F(12,-5)
- 25. STRAIGHT LINE : Question 5 Go to full solution Go to Marker’s Comments Go to Straight Line Menu Go to Main Menu Reveal answer only EXIT In triangle EFG the vertices are E(6,-3), F(12,-5) and G(2,-5). Find (a) the equation of the altitude from vertex E. (b) the equation of the median from vertex F. (c) The point of intersection of the altitude and median. x = 6 (a) x + 8y + 28 = 0 (b) (6,-4.25) (c) X Y G(2,-5) E(6,-3) F(12,-5)
- 28. Markers Comments Begin Solution Continue Solution Question 5(c) Straight Line Menu In triangle EFG the vertices are E(6,-3), F(12,-5) and G(2,-5). Find X Y G(2,-5) E(6,-3) F(12,-5) (c) The point of intersection of the altitude and median. (c) Lines meet when x = 6 & x + 8y + 28 = 0 put x =6 in 2 nd equation 8y + 34 = 0 ie 8y = -34 ie y = -4.25 Point of intersection is (6,-4.25) Back to Home
- 32. HIGHER – ADDITIONAL QUESTION BANK UNIT 1 : Basic Differentiation You have chosen to study: Please choose a question to attempt from the following: 1 2 3 4 5 EXIT Back to Unit 1 Menu
- 33. BASIC DIFFERENTIATION : Question 1 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT Find the equation of the tangent to the curve (x>0) at the point where x = 4.
- 34. BASIC DIFFERENTIATION : Question 1 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT Find the equation of the tangent to the curve (x>0) at the point where x = 4. y = 5 / 4 x – 7
- 35. Markers Comments Begin Solution Continue Solution Question 1 Basic Differentiation Menu Find the equation of the tangent to the curve y = x – 16 x (x>0) at the point where x = 4. NB: a tangent is a line so we need a point of contact and a gradient. Point If x = 4 then y = 4 – 16 4 = 2 – 4 = -2 so (a,b) = (4,-2) Gradient: y = x – 16 x = x 1 / 2 – 16x -1 dy / dx = 1 / 2 x -1 / 2 + 16x -2 = 1 + 16 2 x x 2 If x = 4 then: dy / dx = 1 + 16 2 4 16 = ¼ + 1 = 5 / 4 Continue Solution Back to Home
- 36. Markers Comments Begin Solution Continue Solution Question 1 Basic Differentiation Menu Find the equation of the tangent to the curve y = x – 16 x (x>0) at the point where x = 4. If x = 4 then: dy / dx = 1 + 16 2 4 16 = ¼ + 1 = 5 / 4 Gradient of tangent = gradient of curve so m = 5 / 4 . We now use y – b = m(x – a) this gives us y – (-2) = 5 / 4 (x – 4) or y + 2 = 5 / 4 x – 5 or y = 5 / 4 x – 7 Back to Previous Back to Home
- 39. BASIC DIFFERENTIATION : Question 2 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT Find the coordinates of the point on the curve y = x 2 – 5x + 10 where the tangent to the curve makes an angle of 135 ° with the positive direction of the X-axis.
- 40. BASIC DIFFERENTIATION : Question 2 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT Find the coordinates of the point on the curve y = x 2 – 5x + 10 where the tangent to the curve makes an angle of 135 ° with the positive direction of the X-axis. (2,4)
- 41. Markers Comments Begin Solution Continue Solution Question 2 Basic Differentiation Menu Find the coordinates of the point on the curve y = x 2 – 5x + 10 where the tangent to the curve makes an angle of 135 ° with the positive direction of the X-axis. NB: gradient of line = gradient of curve Line Using gradient = tan we get gradient of line = tan135 ° = -tan45 ° = -1 Curve Gradient of curve = dy / dx = 2x - 5 It now follows that 2x – 5 = -1 Or 2x = 4 Or x = 2 Continue Solution Back to Home
- 42. Markers Comments Begin Solution Continue Solution Question 2 Basic Differentiation Menu Find the coordinates of the point on the curve y = x 2 – 5x + 10 where the tangent to the curve makes an angle of 135 ° with the positive direction of the X-axis. Back to Previous Using y = x 2 – 5x + 10 with x = 2 we get y = 2 2 – (5 X 2) + 10 ie y = 4 So required point is (2,4) Back to Home
- 45. BASIC DIFFERENTIATION : Question 3 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT The graph of y = g(x) is shown here. There is a point of inflection at the origin, a minimum turning point at (p,q) and the graph also cuts the X-axis at r. Make a sketch of the graph of y = g (x). y = g(x) (p,q) r
- 46. BASIC DIFFERENTIATION : Question 3 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT The graph of y = g(x) is shown here. There is a point of inflection at the origin, a minimum turning point at (p,q) and the graph also cuts the X-axis at r. Make a sketch of the graph of y = g (x). y = g(x) (p,q) r y = g (x)
- 47. Markers Comments Begin Solution Continue Solution Question 3 Basic Differentiation Menu Stationary points occur at x = 0 and x = p. (We can ignore r.) We now consider the sign of the gradient either side of 0 and p: new y-values Click for graph y = g(x) (p,q) r Make a sketch of the graph of y = g (x). Back to Home x 0 p g (x) - 0 - 0 +
- 48. Markers Comments Begin Solution Continue Solution Question 3 Basic Differentiation Menu Return to Nature Table y = g(x) (p,q) r Make a sketch of the graph of y = g (x). 0 p y = g (x) This now gives us the following graph Back to Home
- 49. Markers Comments Differentiation Menu Next Comment To sketch the graph of the gradient function: 1 Mark the stationary points on the x axis i.e. Stationary points occur at x = 0 and x = p. Continue Comments Back to Home x y a 0 p y = g (x)
- 50. Markers Comments Differentiation Menu Next Comment 2 For each interval decide if the value of To sketch the graph of the gradient function: 1 Mark the stationary points on the x axis i.e. Stationary points occur at x = 0 and x = p. (We can ignore r.) We now consider the sign of the gradient either side of 0 and p: new y-values Continue Comments + - - x Back to Home x 0 p g (x) - 0 - 0 + y a
- 52. BASIC DIFFERENTIATION : Question 4 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT Here is part of the graph of y = x 3 - 3x 2 - 9x + 2. Find the coordinates of the stationary points and determine their nature algebraically . y = x 3 - 3x 2 - 9x + 2
- 53. BASIC DIFFERENTIATION : Question 4 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT Here is part of the graph of y = x 3 - 3x 2 - 9x + 2. Find the coordinates of the stationary points and determine their nature algebraically . y = x 3 - 3x 2 - 9x + 2 (-1,7) is a maximum TP and (3,-25) is a minimum TP
- 54. BASIC DIFFERENTIATION : Question 4 Return to solution EXIT Here is part of the graph of y = x 3 - 3x 2 - 9x + 2. Find the coordinates of the stationary points and determine their nature algebraically . y = x 3 - 3x 2 - 9x + 2
- 55. Markers Comments Begin Solution Continue Solution Question 4 Basic Differentiation Menu SPs occur where dy / dx = 0 ie 3x 2 – 6x – 9 = 0 ie 3(x 2 – 2x – 3) = 0 ie 3(x – 3)(x + 1) = 0 ie x = -1 or x = 3 Here is part of the graph of y = x 3 - 3x 2 - 9x + 2. Find the coordinates of the stationary points and determine their nature algebraically . Using y = x 3 - 3x 2 - 9x + 2 when x = -1 y = -1 – 3 + 9 + 2 = 7 & when x = 3 y = 27 – 27 - 27 + 2 = -25 So stationary points are at (-1,7) and (3,-25) Continue Solution Back to Home
- 56. Markers Comments Begin Solution Continue Solution Question 4 Basic Differentiation Menu Here is part of the graph of y = x 3 - 3x 2 - 9x + 2. Find the coordinates of the stationary points and determine their nature algebraically . Back to graph We now consider the sign of the gradient either side of -1 and 3. x -1 3 (x + 1) - 0 + + + (x - 3) - - - 0 + dy / dx + 0 - 0 + Hence (-1,7) is a maximum TP and (3,-25) is a minimum TP Back to Home
- 59. BASIC DIFFERENTIATION : Question 5 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT When a company launches a new product its share of the market after x months is calculated by the formula So after 5 months the share is S(5) = 2 / 5 – 4 / 25 = 6 / 25 Find the maximum share of the market that the company can achieve. S(x) = 2 - 4 x x 2 (x 2)
- 60. BASIC DIFFERENTIATION : Question 5 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT When a company launches a new product its share of the market after x months is calculated by the formula So after 5 months the share is S(5) = 2 / 5 – 4 / 25 = 6 / 25 Find the maximum share of the market that the company can achieve. = 1 / 4 S(x) = 2 - 4 x x 2 (x 2)
- 61. Markers Comments Begin Solution Continue Solution Question 5 Basic Differentiation Menu End points S(2) = 1 – 1 = 0 There is no upper limit but as x S(x) 0. Find the maximum share of the market that the company can achieve. When a company launches a new product its share of the market after x months is calculated as: Stationary Points S(x) = 2 - 4 x x 2 = 2x -1 – 4x -2 So S (x) = -2x -2 + 8x -3 = - 2 + 8 x 2 x 3 = 8 - 2 x 3 x 2 Continue Solution Back to Home S(x) = 2 - 4 x x 2 (x 2)
- 62. Markers Comments Continue Solution Question 5 Basic Differentiation Menu Find the maximum share of the market that the company can achieve. When a company launches a new product its share of the market after x months is calculated as: SPs occur where S (x) = 0 8 - 2 x 3 x 2 or 8 = 2 x 3 x 2 ( cross mult!) 8x 2 = 2x 3 8x 2 - 2x 3 = 0 2x 2 (4 – x) = 0 x = 0 or x = 4 NB: x 2 In required interval Continue Solution Go Back to Previous = 0 Back to Home S(x) = 2 - 4 x x 2 (x 2)
- 63. Markers Comments Continue Solution Question 5 Basic Differentiation Menu Find the maximum share of the market that the company can achieve. When a company launches a new product its share of the market after x months is calculated as: Go Back to Previous We now check the gradients either side of X = 4 x 4 S (x) + 0 - S (3.9 ) = 0.00337… S (4.1) = -0.0029… Hence max TP at x = 4 So max share of market = S(4) = 2 / 4 – 4 / 16 = 1 / 2 – 1 / 4 = 1 / 4 Back to Home S(x) = 2 - 4 x x 2 (x 2)
- 67. HIGHER – ADDITIONAL QUESTION BANK UNIT 1 : Recurrence Relations You have chosen to study: Please choose a question to attempt from the following: 1 2 3 EXIT Back to Unit 1 Menu
- 74. RECURRENCE RELATIONS : Question 2 Go to full solution Go to Marker’s Comments Go to Recurrence Relations Menu Go to Main Menu Reveal answer only EXIT Two different recurrence relations are known to have the same limit as n . The first is defined by the formula u n+1 = -5ku n + 3. The second is defined by the formula v n+1 = k 2 v n + 1. Find the value of k and hence this limit.
- 75. RECURRENCE RELATIONS : Question 2 Go to full solution Go to Marker’s Comments Go to Basic Differentiation Menu Go to Main Menu Reveal answer only EXIT Two different recurrence relations are known to have the same limit as n . The first is defined by the formula u n+1 = -5ku n + 3. The second is defined by the formula v n+1 = k 2 v n + 1. Find the value of k and hence this limit. k = 1/3 L = 9/8
- 76. Markers Comments Begin Solution Continue Solution Question 2 Recurrence Relations Menu Continue Solution Two different recurrence relations are known to have the same limit as n . The first is defined by the formula u n+1 = -5ku n + 3. The second is defined by Vn+1 = k 2 v n + 1. Find the value of k and hence this limit. If the limit is L then as n we have u n+1 = u n = L and v n+1 = v n = L First Sequence u n+1 = -5ku n + 3 becomes L + 5kL = 3 L(1 + 5k) = 3 L = 3 . . (1 + 5k) L = -5kL + 3 Second Sequence v n+1 = k 2 v n + 1 becomes L = k 2 L + 1 L - k 2 L = 1 L(1 - k 2 ) = 1 L = 1 . . (1 - k 2 ) Back to Home
- 77. Markers Comments Begin Solution Continue Solution Question 2 Recurrence Relations Menu Continue Solution Two different recurrence relations are known to have the same limit as n . The first is defined by the formula u n+1 = -5ku n + 3. The second is defined by Vn+1 = k 2 v n + 1. Find the value of k and hence this limit. L = 1 . . (1 - k 2 ) It follows that L = 3 . . (1 + 5k) . 3 . = . 1 . . (1 + 5k) (1 – k 2 ) Cross multiply to get 1 + 5k = 3 – 3k 2 This becomes 3k 2 + 5k – 2 = 0 Or (3k – 1)(k + 2) = 0 So k = 1 / 3 or k = -2 Since -1<k<1 then k = 1 / 3 Back to Home
- 78. Markers Comments Begin Solution Continue Solution Question 1 Recurrence Relations Menu Two different recurrence relations are known to have the same limit as n . The first is defined by the formula u n+1 = -5ku n + 3. The second is defined by Vn+1 = k 2 v n + 1. Find the value of k and hence this limit. Since -1<k<1 then k = 1 / 3 Using L = 1 . . (1 - k 2 ) gives us L = . 1 . . (1 – 1 / 9 ) or L = 1 8 / 9 ie L = 9 / 8 Back to Home
- 81. Markers Comments Recurrence Menu Next Comment Since -1<k<1 then k = 1 / 3 Using L = 1 . . (1 - k 2 ) gives us L = . 1 . . (1 – 1 / 9 ) or L = 1 8 / 9 ie L = 9 / 8 Find L from either formula. Back to Home
- 88. HIGHER – ADDITIONAL QUESTION BANK UNIT 1 : Trig Graphs & Equations You have chosen to study: Please choose a question to attempt from the following: 1 2 3 EXIT Back to Unit 1 Menu
- 89. TRIG GRAPHS & EQUATIONS : Question 1 Go to full solution Go to Marker’s Comments Go to Trig Graphs & Equations Menu Go to Main Menu Reveal answer only EXIT This diagram shows the graph of y = acosbx + c. Determine the values of a, b & c. / 2 y = acosbx + c
- 90. TRIG GRAPHS & EQUATIONS : Question 1 Go to full solution Go to Marker’s Comments Go to Trig Graphs & Equations Menu Go to Main Menu Reveal answer only EXIT This diagram shows the graph of y = acosbx + c. Determine the values of a, b & c. a = 3 b = 2 c = -1 / 2 y = acosbx + c
- 91. Markers Comments Begin Solution Continue Solution Question 1 Trig Graphs etc. Menu This diagram shows the graph of y = acosbx + c. Determine the values of a, b & c. a = ½(max – min) = ½(2 – (-4)) = 3 = ½ X 6 Period of graph = so two complete sections between 0 & 2 ie b = 2 For 3cos(…) max = 3 & min = -3. This graph: max = 2 & min = -4. ie 1 less so c = -1 Back to Home / 2 y = acosbx + c
- 93. TRIG GRAPHS & EQUATIONS : Question 2 Go to full solution Go to Marker’s Comments Go to Trig Graphs & Equations Menu Go to Main Menu Reveal answer only EXIT Solve 3tan2 + 1 = 0 ( where 0 < < ).
- 94. TRIG GRAPHS & EQUATIONS : Question 2 Go to full solution Go to Marker’s Comments Go to Trig Graphs & Equations Menu Go to Main Menu Reveal answer only EXIT Solve 3tan2 + 1 = 0 ( where 0 < < ). = 5 / 12 = 11 / 12
- 95. Markers Comments Begin Solution Continue Solution Question 2 Trig Graphs etc. Menu Solve 3tan2 + 1 = 0 ( where 0 < < ). 3tan2 + 1 = 0 3tan2 = -1 tan2 = -1 / 3 Q2 or Q4 tan -1 ( 1 / 3 ) = / 6 Q2: angle = - / 6 so 2 = 5 / 6 ie = 5 / 12 Q4: angle = 2 - / 6 so 2 = 11 / 6 ie = 11 / 12 tan2 repeats every / 2 radians but repeat values are not in interval. Back to Home - + 2 - sin all tan cos 1 2 3 / 6
- 97. TRIG GRAPHS & EQUATIONS : Question 3 Go to full solution Go to Marker’s Comments Go to Trig Graphs & Equations Menu Reveal answer only EXIT The diagram shows a the graph of a sine function from 0 to 2 / 3 . (a) State the equation of the graph. (b) The line y = 2 meets the graph at points P & Q. Find the coordinates of these two points. 2 / 3 P Q y = 2
- 98. TRIG GRAPHS & EQUATIONS : Question 3 Go to full solution Go to Marker’s Comments Go to Trig Graphs & Equations Menu Reveal answer only EXIT The diagram shows a the graph of a sine function from 0 to 2 / 3 . 2 / 3 y = 2 (a) State the equation of the graph. (b) The line y = 2 meets the graph at points P & Q. Find the coordinates of these two points. Graph is y = 4sin3x P is ( / 18 , 2) and Q is ( 5 / 18 , 2). P Q
- 100. (b) The line y = 2 meets the graph at points P & Q. Find the coordinates of these two points. Markers Comments Begin Solution Continue Solution Question 3 Trig Graphs etc. Menu Graph is y = 4sin3x so 4sin3x = 2 or sin3x = 1 / 2 (b) At P & Q y = 4sin3x and y = 2 Q1 or Q2 sin -1 ( 1 / 2 ) = / 6 Q1: angle = / 6 so 3x = / 6 ie x = / 18 Q2: angle = - / 6 so 3x = 5 / 6 ie x = 5 / 18 Back to Home 2 / 3 P Q y = 2 - + 2 - sin all tan cos 1 2 3 / 6 P is ( / 18 , 2) and Q is ( 5 / 18 , 2).
- 103. HIGHER – ADDITIONAL QUESTION BANK UNIT 1 : Functions & Graphs You have chosen to study: Please choose a question to attempt from the following: 1 2 3 4 EXIT Back to Unit 1 Menu
- 104. FUNCTIONS & GRAPHS : Question 1 Go to full solution Go to Marker’s Comments Go to Functions & Graphs Menu Go to Main Menu Reveal answer only EXIT This graph shows the the function y = g(x). Make a sketch of the graph of the function y = 4 – g(-x). y = g(x) -8 12 (-p,q) (u,-v)
- 105. FUNCTIONS & GRAPHS : Question 1 Go to full solution Go to Marker’s Comments Go to Functions & Graphs Menu Go to Main Menu Reveal answer only EXIT This graph shows the the function y = g(x). Make a sketch of the graph of the function y = 4 – g(-x). y = g(x) -8 12 (-p,q) (u,-v) (p,-q+4) (8,4) (-12,4) (0,4) (-u,v+4) y = 4 – g(-x)
- 106. Markers Comments Begin Solution Continue Solution Question 1 Functions & Graphs Menu This graph shows the the function y = g(x). Make a sketch of the graph of the function y = 4 – g(-x). y = 4 – g(-x) = -g(-x) + 4 Reflect in X-axis Reflect in Y-axis Slide 4 up A B C Known Points (-8,0), (-p,q), (0,0), (u,-v), (12,0) (-8,0), (-p,-q), (0,0), (u,v), (12,0) A B (8,0), (p,-q), (0,0), (-u,v), (-12,0) C (8,4), (p,-q+4), (0,4), (-u,v+4), (-12,4) Back to Home y = g(x) -8 12 (-p,q) (u,-v)
- 107. Markers Comments Begin Solution Continue Solution Question 1 Functions & Graphs Menu This graph shows the the function y = g(x). Make a sketch of the graph of the function y = 4 – g(-x). (8,4), (p,-q+4), (0,4), (-u,v+4), (-12,4) Now plot points and draw curve through them. Back to Home y = g(x) -8 12 (-p,q) (u,-v) (p,-q+4) (8,4) (-12,4) (0,4) (-u,v+4) y = 4 – g(-x)
- 111. FUNCTIONS & GRAPHS : Question 2 Go to full solution Go to Marker’s Comments Go to Functions & Graphs Menu Go to Main Menu Reveal answer only EXIT This graph shows the the function y = a x . Make sketches of the graphs of the functions (I) y = a (x+2) (II) y = 2a x - 3 y = a x (1,a)
- 112. FUNCTIONS & GRAPHS : Question 2 Go to full solution Go to Marker’s Comments Go to Functions & Graphs Menu Reveal answer only EXIT This graph shows the the function y = a x . Make sketches of the graphs of the functions (I) y = a (x+2) (II) y = 2a x - 3 ANSWER TO PART (I) ANSWER to PART (II) (-1,a) (-2,1) y = a (x+2) y = a x y = 2a x - 3 y = a x (0,-1) (1,2a-3)
- 113. Markers Comments Begin Solution Continue Solution Question 2 Functions & Graphs Menu Make sketches of the graphs of the functions (I) y = a (x+2) (I) y = a (x+2) f(x) = a x so a (x+2) = f(x+2) move f(x) 2 to left (0,1) ( -2 ,1) & (1,a) ( -1 ,a) Back to Home y = a x (1,a) (-1,a) (-2,1) y = a (x+2) y = a x
- 114. Markers Comments Begin Solution Continue Solution Question 2 Functions & Graphs Menu Make sketches of the graphs of the functions (II) y = 2a x - 3 (II) y = 2a x - 3 f(x) = a x so 2a x - 3 = 2f(x) - 3 double y-coords slide 3 down (0,1) (0, 2 ) (0 ,-1 ) (1,a) (1, 2a ) (1, 2a-3 ) Back to Home y = a x (1,a) y = 2a x - 3 y = a x (0,-1) (1,2a-3)
- 119. FUNCTIONS & GRAPHS : Question 3 Go to full solution Go to Marker’s Comments Go to Functions & Graphs Go to Main Menu Reveal answer only EXIT Two functions f and g are defined on the set of real numbers by the formulae f(x) = 2x - 1 and g(x) = x 2 . (b) Hence show that the equation g(f(x)) = f(g(x)) has only one real solution. (a) Find formulae for (i) f(g(x)) (ii) g(f(x)) .
- 120. FUNCTIONS & GRAPHS : Question 3 Go to full solution Go to Marker’s Comments Go to Functions & Graphs Go to Main Menu Reveal answer only EXIT Two functions f and g are defined on the set of real numbers by the formulae f(x) = 2x - 1 and g(x) = x 2 . (b) Hence show that the equation g(f(x)) = f(g(x)) has only one real solution. (a) Find formulae for (i) f(g(x)) (ii) g(f(x)) . = 2x 2 - 1 (a) (i) = 4x 2 – 4x + 1 (ii)
- 125. FUNCTIONS & GRAPHS : Question 4 Go to full solution Go to Marker’s Comments Go to Functions & Graphs Menu Go to Main Menu Reveal answer only EXIT A function g is defined by the formula g(x) = . 2 (x 1) (x – 1) (a) Find a formula for h(x) = g(g(x)) in its simplest form. (b) State a suitable domain for h.
- 126. FUNCTIONS & GRAPHS : Question 4 Go to full solution Go to Marker’s Comments Go to Functions & Graphs Menu Go to Main Menu Reveal answer only EXIT A function g is defined by the formula g(x) = . 2 (x 1) (x – 1) (a) Find a formula for h(x) = g(g(x)) in its simplest form. (b) State a suitable domain for h. h(x) = (2x - 2) . . (3 – x) Domain = {x R: x 3}
- 131. HIGHER – ADDITIONAL QUESTION BANK UNIT 2 : Integration Polynomials The Circle Addition Formulae Quadratics EXIT
- 132. HIGHER – ADDITIONAL QUESTION BANK UNIT 2 : Polynomials You have chosen to study: Please choose a question to attempt from the following: 1 2 3 4 EXIT Back to Unit 2 Menu
- 133. POLYNOMIALS : Question 1 Go to full solution Go to Marker’s Comments Go to Polynomial Menu Go to Main Menu Reveal answer only EXIT Show that x = 3 is a root of the equation x 3 + 3x 2 – 10x – 24 = 0 . Hence find the other roots.
- 134. POLYNOMIALS : Question 1 Go to full solution Go to Marker’s Comments Go to Polynomial Menu Go to Main Menu Reveal answer only EXIT Show that x = 3 is a root of the equation x 3 + 3x 2 – 10x – 24 = 0 . Hence find the other roots. other roots are x = -4 & x = -2
- 135. Markers Comments Begin Solution Continue Solution Question 1 Polynomial Menu Back to Home Show that x = 3 is a root of the equation x 3 + 3x 2 – 10x – 24 = 0 . Hence find the other roots. Using the nested method - coefficients are 1, 3, -10, -24 f(3) = 3 1 3 -10 -24 3 18 24 1 6 8 0 f(3) = 0 so x = 3 is a root. Also (x – 3) is a factor. Other factor: x 2 + 6x + 8 or (x + 4)(x + 2) If (x + 4)(x + 2) = 0 then x = -4 or x = -2 Hence other roots are x = -4 & x = -2
- 137. Given that (x + 4) is a factor of the polynomial f(x) = 3x 3 + 8x 2 + kx + 4 find the value of k. Hence solve the equation 3x 3 + 8x 2 + kx + 4 = 0 for this value of k. POLYNOMIALS : Question 2 Go to full solution Go to Marker’s Comments Go to Polynomial Menu Go to Main Menu Reveal answer only EXIT
- 138. Given that (x + 4) is a factor of the polynomial f(x) = 3x 3 + 8x 2 + kx + 4 find the value of k. Hence solve the equation 3x 3 + 8x 2 + kx + 4 = 0 for this value of k. POLYNOMIALS : Question 2 Go to full solution Go to Marker’s Comments Go to Polynomial Menu Go to Main Menu Reveal answer only EXIT k = -15 So full solution of equation is x = -4 or x = 1 / 3 or x = 1
- 139. Markers Comments Begin Solution Continue Solution Question 2 Polynomial Menu Back to Home Given that (x + 4) is a factor of the polynomial f(x) = 3x 3 + 8x 2 + kx + 4 find the value of k. Hence solve the equation 3x 3 + 8x 2 + kx + 4 = 0 for this value of k. Since (x + 4) a factor then f(-4) = 0 . Now using the nested method - coefficients are 3, 8, k, 4 f(-4) = -4 3 8 k 4 -12 16 (-4k – 64) 3 -4 (k + 16) (-4k – 60 ) Since -4k – 60 = 0 then -4k = 60 so k = -15
- 140. Markers Comments Begin Solution Continue Solution Question 2 Polynomial Menu Back to Home Given that (x + 4) is a factor of the polynomial f(x) = 3x 3 + 8x 2 + kx + 4 find the value of k. Hence solve the equation 3x 3 + 8x 2 + kx + 4 = 0 for this value of k. If k = -15 then we now have f(-4) = -4 3 8 -15 4 -12 16 -4 Other factor is 3x 2 – 4x + 1 or (3x - 1)(x – 1) 3 -4 1 0 If (3x - 1)(x – 1) = 0 then x = 1 / 3 or x = 1 So full solution of equation is: x = -4 or x = 1 / 3 or x = 1
- 144. POLYNOMIALS : Question 3 Go to full solution Go to Marker’s Comments Go to Polynomial Menu Go to Main Menu Reveal answer only EXIT Given that f(x) = 6x 3 + 13x 2 - 4 show that (x + 2) is a factor of f(x). Hence express f(x) in its fully factorised form.
- 145. POLYNOMIALS : Question 3 Go to full solution Go to Marker’s Comments Go to Polynomial Menu Go to Main Menu Reveal answer only EXIT Given that f(x) = 6x 3 + 13x 2 - 4 show that (x + 2) is a factor of f(x). Hence express f(x) in its fully factorised form. 6x 3 + 13x 2 - 4 = (3x + 2)(2x - 1)(x + 2)
- 146. Markers Comments Begin Solution Continue Solution Question 3 Polynomial Menu Back to Home Given that f(x) = 6x 3 + 13x 2 - 4 show that (x + 2) is a factor of f(x). Hence express f(x) in its fully factorised form. Using the nested method - coefficients are 6, 13, 0, -4 f(-2) = -2 6 13 0 -4 6 -12 1 -2 -2 4 0 f(-2) = 0 so (x + 2) is a factor
- 147. Markers Comments Begin Solution Continue Solution Question 3 Polynomial Menu Back to Home Given that f(x) = 6x 3 + 13x 2 - 4 show that (x + 2) is a factor of f(x). Hence express f(x) in its fully factorised form. Using the nested method - coefficients are 6, 13, 0, -4 f(-2) = -2 6 13 0 -4 6 -12 1 -2 -2 4 0 Other factor is 6x 2 + x – 2 or (3x + 2)(2x - 1) Hence 6x 3 + 13x 2 - 4 = (3x + 2)(2x - 1)(x + 2)
- 154. Markers Comments Begin Solution Continue Solution Question 4 Polynomial Menu Back to Home y = -x 3 + 6x 2 – 3x – 10 (b) The tangent & curve meet whenever y = -3x + 22 and y = -x 3 + 6x 2 – 3x – 10 ie -3x + 22 = -x 3 + 6x 2 – 3x – 10 or x 3 - 6x 2 + 32 = 0 (b)Hence find the coordinates of Q – the point where the bypass rejoins the original road. We already know that x = 4 is one solution to this so using the nested method we get ….. f(4) = 4 1 -6 0 32 4 -8 -32 1 -2 -8 0 Other factor is x 2 – 2x - 8 PQ is y = -3x + 22 P Q y = -x 3 + 6x 2 – 3x – 10 4
- 155. Markers Comments Begin Solution Continue Solution Question 4 Polynomial Menu Back to Home y = -x 3 + 6x 2 – 3x – 10 (b) The (b)Hence find the coordinates of Q – the point where the bypass rejoins the original road. other factor is x 2 – 2x - 8 = (x – 4)(x + 2) Solving (x – 4)(x + 2) = 0 we get x = 4 or x = -2 It now follows that Q has an x-coordinate of -2 Using y = -3x + 22 if x = -2 then y = 6 + 22 = 28 Hence Q is (-2,28) PQ is y = -3x + 22 P Q y = -x 3 + 6x 2 – 3x – 10 4
- 160. HIGHER – ADDITIONAL QUESTION BANK UNIT 2 : Quadratics You have chosen to study: Please choose a question to attempt from the following: 1 2 3 4 5 EXIT Back to Unit 2 Menu 6
- 161. QUADRATICS : Question 1 Go to full solution Go to Marker’s Comments Go to Quadratics Menu Go to Main Menu Reveal answer only EXIT (a) Express f(x) = x 2 – 8x + 21 in the form (x – a) 2 + b. (b) Hence, or otherwise, sketch the graph of y = f(x).
- 162. QUADRATICS : Question 1 Go to full solution Go to Marker’s Comments Go to Quadratics Menu Go to Main Menu Reveal answer only EXIT (a) Express f(x) = x 2 – 8x + 21 in the form (x – a) 2 + b. (b) Hence, or otherwise, sketch the graph of y = f(x). y = x 2 – 8x + 21 (4,5) (b) = (x – 4) 2 + 5 (a)
- 175. QUADRATICS : Question 3 Go to full solution Go to Marker’s Comments Go to Quadratics Menu Go to Main Menu Reveal answer only EXIT For what value(s) of k does the equation 4x 2 – kx + (k + 5) = 0 have equal roots.
- 176. QUADRATICS : Question 3 Go to full solution Go to Marker’s Comments Go to Quadratics Menu Go to Main Menu Reveal answer only EXIT For what value(s) of k does the equation 4x 2 – kx + (k + 5) = 0 have equal roots. k = -4 or k = 20
- 177. Markers Comments Begin Solution Continue Solution Question 3 Quadratics Menu Back to Home For what value(s) of k does the equation 4x 2 – kx + (k + 5) = 0 have equal roots. Let 4x 2 – kx + (k + 5) = ax 2 + bx + c then a = 4, b = -k & c = (k + 5) For equal roots we need discriminant = 0 ie b 2 – 4ac = 0 (- k ) 2 – (4 X 4 X ( k + 5 )) = 0 k 2 – 16(k + 5) = 0 k 2 – 16k - 80 = 0 (k + 4)(k – 20) = 0 k = -4 or k = 20
- 180. QUADRATICS : Question 4 Go to full solution Go to Marker’s Comments Go to Quadratics Menu Go to Main Menu Reveal answer only EXIT The equation of a parabola is f(x) = px 2 + 5x – 2p . Prove that the equation f(x) = 0 always has two distinct roots.
- 181. QUADRATICS : Question 4 Go to full solution Go to Marker’s Comments Go to Quadratics Menu Go to Main Menu Reveal answer only EXIT The equation of a parabola is f(x) = px 2 + 5x – 2p . Prove that the equation f(x) = 0 always has two distinct roots. discriminant = b 2 – 4ac = 8p 2 + 25 Since p 2 0 for all values of p then 8p 2 + 25 > 0. The discriminant is always positive so there are always two distinct roots.
- 182. Markers Comments Begin Solution Continue Solution Question 4 Quadratics Menu Back to Home The equation of a parabola is f(x) = px 2 + 5x – 2p . Prove that the equation f(x) = 0 always has two distinct roots. Let px 2 + 5x – 2p = ax 2 + bx + c then a = p, b = 5 & c = -2p . So discriminant = b 2 – 4ac = 5 2 – (4 X p X (- 2p )) = 25 – (-8p 2 ) = 8p 2 + 25 Since p 2 0 for all values of p then 8p 2 + 25 > 0. The discriminant is always positive so there are always two distinct roots.
- 186. QUADRATICS : Question 5 Go to full solution Go to Marker’s Comments Go to Quadratics Menu Go to Main Menu Reveal answer only EXIT Given that the roots of 3x(x + p) = 4p(x – 1) are equal then show that p = 0 or p = 48.
- 187. QUADRATICS : Question 5 Go to full solution Go to Marker’s Comments Go to Quadratics Menu Go to Main Menu Reveal answer only EXIT Given that the roots of 3x(x + p) = 4p(x – 1) are equal then show that p = 0 or p = 48. For equal roots we need discriminant = 0 p(p - 48) = 0 ie p = 0 or p = 48
- 188. Markers Comments Begin Solution Continue Solution Question 5 Quadratics Menu Back to Home Given that the roots of 3x(x + p) = 4p(x – 1) are equal then show that p = 0 or p = 48. Rearranging 3x(x + p) = 4p(x – 1) 3x 2 + 3px = 4px - 4p 3x 2 - px + 4p = 0 Let 3x 2 - px + 4p = ax 2 + bx + c then a = 3, b = -p & c = 4p For equal roots we need discriminant = 0 ie b 2 – 4ac = 0 (- p ) 2 - (4 X 3 X 4p ) = 0 p 2 - 48p = 0 p(p - 48) = 0 ie p = 0 or p = 48
- 192. QUADRATICS : Question 6 Go to full solution Go to Marker’s Comms Go to Quadratics Menu Go to Main Menu Reveal answer only EXIT The diagram below shows the parabola y = -2x 2 + 3x + 2 and the line x + y – 4 = 0. Prove that the line is a tangent to the curve. y = -2x 2 + 3x + 2 x + y – 4 = 0
- 193. QUADRATICS : Question 6 Go to full solution Go to Marker’s Comms Go to Quadratics Menu Go to Main Menu Reveal answer only EXIT The diagram below shows the parabola y = -2x 2 + 3x + 2 and the line x + y – 4 = 0. Prove that the line is a tangent to the curve. Since the discriminant = 0 then there is only one solution to the equation so only one point of contact and it follows that the line is a tangent. y = -2x 2 + 3x + 2 x + y – 4 = 0
- 194. Markers Comments Begin Solution Continue Solution Question 6 Quadratics Menu Back to Home y = -2x 2 + 3x + 2 x + y – 4 = 0 Prove that the line is a tangent. Linear equation can be changed from x + y – 4 = 0 to y = -x + 4 . The line and curve meet when y = -x + 4 and y = -2x 2 + 3x + 2 . So -x + 4 = -2x 2 + 3x + 2 Or 2x 2 - 4x + 2 = 0 Let 2x 2 - 4x + 2 = ax 2 + bx + c then a = 2, b = -4 & c = 2.
- 195. Markers Comments Begin Solution Continue Solution Question 6 Quadratics Menu Back to Home y = -2x 2 + 3x + 2 x + y – 4 = 0 Prove that the line is a tangent. then a = 2, b = -4 & c = 2. So discriminant = b 2 – 4ac = (- 4 ) 2 – (4 X 2 X 2 ) = 16 - 16 = 0 Since the discriminant = 0 then there is only one solution to the equation so only one point of contact and it follows that the line is a tangent.
- 198. HIGHER – ADDITIONAL QUESTION BANK UNIT 2 : Integration You have chosen to study: Please choose a question to attempt from the following: 1 2 3 4 5 EXIT Back to Unit 2 Menu
- 199. Show that the shaded area is given by 1 / 3 k 3 – 4k 2 + 18k - 27 INTEGRATION : Question 1 Go to full solution Go to Marker’s Comments Go to Integration Menu Go to Main Menu Reveal answer only EXIT The diagram below shows the curve y = x 2 - 8x + 18 and the lines x = 3 and x = k. y = x 2 - 8x + 18 x = 3 x = k
- 200. The diagram below shows the curve y = x 2 - 8x + 18 and the lines x = 3 and x = k. Show that the shaded area is given by 1 / 3 k 3 – 4k 2 + 18k - 27 INTEGRATION : Question 1 Go to full solution Go to Marker’s Comments Go to Integration Menu Go to Main Menu Reveal answer only EXIT = 1 / 3 k 3 – 4k 2 + 18k – 27 as required. y = x 2 - 8x + 18 x = 3 x = k Area = (x 2 - 8x + 18) dx 3 k
- 201. Markers Comments Begin Solution Continue Solution Question 1 Integration Menu Back to Home The diagram shows the curve y = x 2 - 8x + 18 and the lines x = 3 and x = k . Show that the shaded area is given by 1 / 3 k 3 – 4k 2 + 18k - 27 = ( 1 / 3 k 3 – 4k 2 + 18k) – (( 1 / 3 X 27) – (4 X 9) + 54) = 1 / 3 k 3 – 4k 2 + 18k – 27 as required. Area = (x 2 - 8x + 18) dx 3 k = x 3 - 8x 2 + 18x [ ] 3 2 k 3 = 1 / 3 x 3 – 4x 2 + 18x [ ] k 3
- 204. INTEGRATION : Question 2 Go to full solution Go to Marker’s Comments Go to Integration Menu Go to Main Menu Reveal answer only EXIT Given that dy / dx = 12x 2 – 6x and the curve y = f(x) passes through the point (2,15) then find the equation of the curve y = f(x).
- 205. INTEGRATION : Question 2 Go to full solution Go to Marker’s Comments Go to Integration Menu Go to Main Menu Reveal answer only EXIT Given that dy / dx = 12x 2 – 6x and the curve y = f(x) passes through the point (2,15) then find the equation of the curve y = f(x). Equation of curve is y = 4x 3 – 3x 2 - 5
- 206. Markers Comments Begin Solution Continue Solution Question 2 Integration Menu Back to Home Given that dy / dx = 12x 2 – 6x and the curve y = f(x) passes through the point (2,15) then find the equation of the curve y = f(x). dy / dx = 12x 2 – 6x So = 4x 3 – 3x 2 + C Substituting (2,15) into y = 4x 3 – 3x 2 + C We get 15 = (4 X 8) – (3 X 4) + C So C + 20 = 15 ie C = -5 Equation of curve is y = 4x 3 – 3x 2 - 5 = 12x 3 – 6x 2 + C 3 2
- 209. INTEGRATION : Question 3 Go to full solution Go to Marker’s Comments Go to Integration Menu Go to Main Menu Reveal answer only EXIT Find x 2 - 4 2x x dx
- 210. INTEGRATION : Question 3 Go to full solution Go to Marker’s Comments Go to Integration Menu Go to Main Menu Reveal answer only EXIT Find x 2 - 4 2x x dx = x x + 4 + C 3 x
- 211. Markers Comments Begin Solution Continue Solution Question 3 Integration Menu Back to Home = 2 / 3 X 1 / 2 x 3 / 2 - (-2) X 2x -1 / 2 + C = 1 / 3 x 3 / 2 + 4x -1 / 2 + C Find x 2 - 4 2x x dx x 2 - 4 2x x dx = x 2 - 4 2x 3 / 2 2x 3 / 2 dx = 1 / 2 x 1 / 2 - 2x -3 / 2 dx = x x + 4 + C 3 x
- 213. INTEGRATION : Question 4 Go to full solution Go to Marker’s Comments Go to Integration Menu Go to Main Menu Reveal answer only EXIT 1 2 ( ) Evaluate x 2 - 2 2 dx x
- 214. INTEGRATION : Question 4 Go to full solution Go to Marker’s Comments Go to Integration Menu Go to Main Menu Reveal answer only EXIT = 2 1 / 5 1 2 ( ) Evaluate x 2 - 2 2 dx x
- 215. Markers Comments Begin Solution Continue Solution Question 4 Integration Menu Back to Home = 2 1 / 5 = ( 32 / 5 - 8 - 2) - ( 1 / 5 - 2 - 4) 1 2 ( ) Evaluate x 2 - 2 2 dx x 1 2 ( ) x 2 - 2 2 dx x ( ) = x 4 - 4x + 4 dx x 2 1 2 ( ) = x 4 - 4x + 4x -2 dx 1 2 [ ] = x 5 - 4x 2 + 4x -1 5 2 -1 1 2 = x 5 - 2x 2 - 4 5 x [ ] 2 1
- 216. Markers Comments Int