Successive differentiation

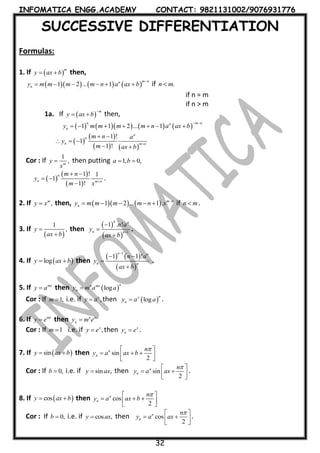
INFOMATICA ENGG.ACADEMY CONTACT: 9821131002/9076931776
32
SUCCESSIVE DIFFERENTIATION
Formulas:
1. If  
m
y ax b  then,
      1 2 ... 1
m nn
ny m m m m n a ax b

      if .n m
if n = m
if n > m
1a. If  
m
y ax b

  then,
        1 1 2 ... 1
n m nn
ny m m m m n a ax b
 
      
 
 
   
1 !
1
1 !
n
n
n m n
m n a
y
m ax b

 
  
 
Cor : If
1
,m
y
x
 then putting 1, 0,a b 
 
 
 
1 ! 1
1
1 !
n
n m n
m n
y
m x 
 
 

.
2. If ,m
y x then,     1 2 ... 1 . m n
ny m m m m n x 
     if n m .
3. If
 
1
,y
ax b


then
 
 
1
1 . !
n n
n n
n a
y
ax b




.
4. If  logy ax b  then
   
 
1
1 1 !
n n
n n
n a
y
ax b

 


.
5. If mx
y a then  log
nn mx
ny m a a
Cor : If 1,m  i.e. if ,x
y a then  log
nx
ny a a .
6. If mx
y e then n mx
ny m e
Cor : If 1m  i.e. if ,x
y e then x
ny e .
7. If  siny ax b  then sin
2
n
n
n
y a ax b
 
    
Cor : If 0,b  i.e. if sin ,y ax then sin
2
n
n
n
y a ax
 
   
.
8. If  cosy ax b  then cos
2
n
n
n
y a ax b
 
    
Cor : If 0,b  i.e. if cos ,y ax then cos
2
n
n
n
y a ax
 
   
.

33
9. If  sinax
y e bx c  then  sinn ax
ny r e bx c n  
where 2 2
r a b  and 1
tan
b
a
   
  
 
Cor : If 0,c  i.e. if sinax
y e bx , then  sinn ax
ny r e bx n  .
10. If  cosax
y e bx c  then  cosn ax
ny r e bx c n  
Cor : If 0,c  i.e. if cosax
y e bx , then  cosn ax
ny r e bx n  .
11. If  sinx
y k bx c  then  sinn x
ny r k bx c n  
where  
2 2
logr k b  and 1
tan
log
b
k
   
  
 
.
12. If  cosx
y k bx c  then  cosn x
ny r k bx c n  
where  
2 2
logr k b  and 1
tan
log
b
k
   
  
 
.

34
Type 1 : nth Derivative Of Algebraic Functions
Questions Answers
1. 2 2
x
x a
 
   
1 1
1 ! 1 1
2
n
n n
n
x a x a
 
 
 
   
2. 4 4
1
x a
 
   
1 13
1 ! 1 1
4
n
n n
n
a x a x a
 
 
 
   
 
   
1 13
1 ! 1 1
4 ! ! !
n
n n
n
a x a x a
 
 
  
   
3.
  
4
1 2
x
x x     
2 16 1
3 7
2 1
y x x
x x
    
 
 
   
1 1
16 1
1 !
2 1
n
n n n
y n
x x
 
 
   
   
for 3n 
4.
2
4
1
x
x
 
   
1 1
1 ! 1 1
4 1 1
n
n n
n
x x
 
 
 
   
 
   
1 1
1 ! 1 1
4!
n
n n
n
x i x i
 
 
  
   
5. 2
1
6 5 1x x 
 
   
1 1
1 1
2 3
1 !
2 1 3 1
n n
n
n n
n
x x
 
 
 
  
   
6. 2
1
4
x
x


 
   
1 1
3 1 1 1
1 ! . .
4 42 2
 
 
  
   
n
n n
n
x x
7. 3 2
6 11 6
x
x x x  
 
     
1 1 1
1 2 3
1 !
2 1 2 2 3
n
n n n
n
x x x
  
 
   
    
8.
  
2
2
4
2 3 1
x
x x

 
(M.U.1992)
 
 
   
 
1 2
1 !2 1 1 !
2 3 1
n nn
n n
n n
x x
 
  

 
9. 2
9
x
x 
 
   
1 1
1 ! 1 1
2 3 3
n
n n
n
x i x i
 
 
 
   

35
10.
  
2
1 2 3
x
x x 
 
   
1 1
1 ! 2 9.2
10 1 2 3
n n
n n
n
x x
 
 
 
   
11. 2
1 4
x
x
(M.U.1983)
   
 
 
 
1 1
1 ! 2 2
4 1 2 1 2
n n n
n n
n
x x
 
  
 
   
12.
 
5
1
x
x 
   
 
 5
1 3 !
4
4! 1
n
n
n
x n
x

 


13.
   
2
4
1 1
x
x x 
(M.U.1983, 2002, 04)  
 
 
   
1 2 1
2 1 !! !
1
1 1 1
n
n n n
nn n
x x x
  
 
   
    
14.
  
2
1
3 2 3x x 
(M.U.1995)
 
2
9 1 3 1 1 1
. . .
49 3 2 49 3 7 3
y
x x x
  
  
 
 
 
 
   
 
1 1 2
1 . !3 1 . ! 1 . 1 !9 3 1
.
49 49 73 2 3 3
n n nn
n n n n
n n n
y
x x x
  
   
  
  
15.
2
3 2
4 1
2 2
x x
x x x
 
  
(M.U.1984)  
     
1 1 1
1 1 1
1 . !
1 1 2
n
n n n
n
x x x
  
 
   
    
16. If
 
 
1
log ,
1
x
y x
x



prove that    
   
1 2 !
1 1
n
n n n
x n x n
y n
x x
  
    
   
Type 2 : nth Derivative Of Trigonometric Functions
1. sin cos3x x Ans :
1
. 4 sin 4 2 sin 2
2 2 2
n nn n
x x
     
      
    
2. sin2 sin3 cos4x x x (M.U.2004)
Ans :        
1
5 cos 5 / 2 3 cos 3 / 2 9 cos 9 / 2 cos / 2
4
n n n
x n x n x n x n           
3. sin2 sin3 sin4x x x
Ans :        
1
5 sin 5 / 2 3 sin 3 / 2 sin / 2 9 sin 9 / 2
4
n n n
x n x n x n x n           
4. 3
sin 3x Ans :    
3 1
.3 sin 3 / 2 .9 sin 9 / 2
4 4
n n
x n x n   
5. 4
sin x Ans :
3 1 1
cos2 cos4
8 2 8
y x x  
   
1 1
.2 cos 2 / 2 4 cos 4 / 2
2 8
n n
ny x n x n     

36
6. 4
cos x Ans :
3 1 1
cos2 cos4
8 2 8
y x x  
   
1 1
.2 cos 2 / 2 4 cos 4 / 2
2 8
n n
ny x n x n    
7. 2 3
cos sinx x Ans :      
1
2sin / 2 3 sin 3 / 2 5 sin 5 / 2
16
n n
y x n x n x n         
8. cos2 cosx
e x x Ans :    /2 1 /21
10 cos 3 tan 3 2 cos / 4
2
x n n
e x n x n
    
9. 5
cos cos3x
e x x (M.U.2007) Ans : 5 /2 1 /2 11 4 2
41 cos 4 tan 29 cos 2 tan
2 5 5
x n n
e x n x n        
         
       
10. 2
sin cosx
e x x Ans :      3/2 1 /2 11 1
10 cos 3 tan 3 2 cos tan 1
4 4
x n x
e x n e x n 
   
11. 2
cos cosx
e x x Ans :    /2 1 /2
10 cos 3 tan 3 2 cos / 4
4
x
n ne
x n x n
    
12. 2
cos sinx
e x x Ans :    /2 1 /2
10 sin 3 tan 3 2 sin / 4
4
x
n ne
x n x n
    
13. cos2 sinax
e x x Ans :    
/2 /22 1 2 13 1
9 sin 3 tan 1 sin tan
2
ax
n ne
a x n a x n
a a
        
           
       
14. 2
2 sin cosx
x x Ans :    1 1 2 2
1 1
2 cos 3 2 cos
4 4
n x n x
r x n r x n    
where    
2 2 1
1 1log2 3 , tan 3/ log2 ,r  
  
   
2 2 1
2 2log2 1 , tan 1/ log2r  
  
15. If cosh 2y x prove that 2 sinh 2n
ny x if n is odd and 2 cosh 2n
ny x if n is even.
(M.U.2002) .
Type 3 : nth Derivative Using De Moivre’s Theorem
1. If 2
1
,
1
y
x


prove that    1
1 . sin sin 1
n n
ny n n 
   where  1
tan 1/ .x 

2. If 2
,
1
x
y
x


1 . !sin cos 1
n n
ny n n 
   where  1
tan 1/ .x 

3. If  1
tan / ,y x a
 prove that    
1
1 1 ! sin sin
n n n
ny n a n 
 
   where  1
tan 1/ .x 

4. If 1
2
2
tan ,
1
x
y
x
  
  
 
1
2. 1 1 !sin sin
n n
ny n n 

   where  1
tan 1/ .x 

5. If 1
2
2
sin ,
1
x
y
x
  
  
 
1
2. 1 1 !sin sin
n n
ny n n 

   where  1
tan 1/ .x 

6. If
2
1
2
1
sec ,
1
x
y
x
  
  
 
1
2 1 1 !sin sin
n n
ny n n 

   where  1
tan 1/ .x 

7. If 1
tan ,y x x
 find ny (M.U.1998)
Ans :          
1 2 1
1 1 !sin cos 1 2 !sin sin 1
n nn n
n n n   
  
      where  1
tan 1/ .x 

8. If 7
sin ,y x find ny
Ans :
1
7 sin 7 7.5 sin 5 21.3 sin 3 35sin
64 2 2 2 2
n n n
n
n n n n
y x x x x
           
                
        
9. If 4 3
sin cos ,y x x find ny

37
Ans :
1
7 cos 7 cos 5 3.3 cos 3 3cos
64 2 2 2 2
n n
n
n n n n
y x x x x
           
               
        
10. If 5
sin ,y x find ny Ans :
1
5 sin 5 5.3 sin 3 10sin
16 2 2 2
n n
n
n n n
y x x x
        
           
      
.
Type 4 : Leibnitz’s Theorem
0 1 1 1 2 2 2 .....n n n
n n n ny C u v C u v C u v     .......n n
r n r r n nC u v C uv  
1. If  1
cos sin ,y m x
 prove that      2 2 2
2 11 2 1 0.n n nx y n xy m n y      
Hence, obtain  0 .ny (M.U.2008)
Soln
: We have  1
1 2
1
sin sin . .
1
y m x m
x

 

 2 1
11 . sin sin .x y m m x
   
Differentiating again, we get
 2 1
2 2 2
1
1 cos sin .
1 1
xy
x y m m x m
x x

   
 
 2 2
2 11 0.x y xy m y     ………………………(1)
Applying Leibnitz’s Theorem to each term,
   
 
 2
2 1
1
1 2 2
2!
n n n
n n
x y n x y y 

    
2
1 0.n n nxy ny m y   
   2
2 11 2 1n n nx y nxy n n y    
2
1 0.n n nxy ny m y   
     2 2 2
2 11 2 1 0n n nx y n xy m n y        ………………..(2)
Putting 0x  in
     1
cos sin , 0 cos 0 1y m x y
  
Putting 0x  in
 1
1 2
1
sin sin ,
1
y m x
x



we get    1 0 sin 0 0y  
Putting 0x  in (1), we get    2 2
2 0 0y m y m   
Putting 0x  in (2),
     2 2
2 0 0 ...................(3)  n ny n m y
Putting 1,3,5........x  in (3), we get,
     
     
2 2
3 1
2 2
5 3
0 1 0 0
0 3 0 0
y m y
y m y
  
  
 0 0ny  if n is odd
Putting 2,4,6.......n  in (3), we get.

38
        
         
       
2 2 2 2 2
4 2
2 2 2 2 2 2 2
6 4
2 2 2 2 2 2 2
0 2 0 2
0 4 0 4 2
0 .......... 4 2 .n
y m y m m
y m y m m m
y n m m m m
    
     
     
2. If
1
sin 1
sin log ,m x
y e or x y
m
   
    
  
prove that      2 2 2
2 11 2 1 0.n n nx y n x y n m y      
Soln
: We have,
1
sin
1 2 2
1
.
1 1
m x my
y e m
x x
  
  
  
2
11 .x y my  
Differentiating again, w.r.t., x
2
2 1
2 12 2
1 .
1 1
   
 
xy m y
x y my
x x
 2 2
2 11 x y xy m y   
Applying Leibnitz’s Theorem, to each term,
   
 
 2
2 1
1
1 2 2
2!
n n n
n n
x y n x y y 

    
  2
1n n nxy ny m y  
     2 2 2
2 11 2 1 0.n n nx y n x y n m y       
3. If
1
sin
,

 a x
y e prove that
       
22 2 2 2 2 2 2
2 0 2 4 6 ........ 2 2my a a a a a m      
 
       
22 2 2 2 2 2 2 2
2 1 0 1 3 5 .......... 2 1my a a a a a m
      
 
(M.U.1985)
Soln
: We have
1
sin
,a x
y e

 ……………………………………(1)
Replacing m by a in the above example, we get,
1 2
1
ay
y
x


…………………………………..(2)
 2 2
2 11 x y xy a y   ……………………………….(3)
     2 2 2
2 11 2 1 0n n nx y n xy n a y       ………………………….(4)
Putting 0,x  in (1), we get,
  0
0 1y e 
   1 0 0y a y a 
   2 2
2 0 0y a y a 
Putting 0x  in (4), we get,
     2 2
2 0 0n ny n a y   …………………………………….(5)
Putting 1,n  in (5),
       2 2 2 2
3 10 1 0 1y a y a a   
Putting 2n  in (5),

39
       2 2 2 2 2
4 20 2 0 2y a y a a   
        2 2 2 2 2 2
5 30 3 0 1 3y a y a a a     …………………(6)
        2 2 2 2 2 2 2
6 40 4 0 2 4y a y a a a     ………………………(7)
Generalising from (6) and (7), we get
       
       
22 2 2 2 2 2 2
2 1
22 2 2 2 2 2 2 2
2
0 1 3 5 ......... 2 1
0 2 4 6 .......... 2 2
m
m
y a a a a a m
y a a a a a m

      
 
      
 
4. If   1,x y  prove that
       
2 21 2 2
1 2! . . ..... 1         
n
nn n n n n n n n
nn
d
x y n y C y x C y x x
dx
(M.U.2006)
Soln
: Since 1x y  1y x  
   1
n n
nn n n
n n
d d
x y x x
dx dx
  
 
Let  , 1
nn n
u x v x y   
Then    .
n n
n n
n n
d d
x y u v
dx dx

Now
 
    
   
 
     
    
 
1
1
2
2
1
2 2
2
1 1
1
2 2
2
2
1
.....................................................
1 ..... 3 2 !
!
1 ..... 3 .
2
1 ...3.2.1 !
1 1 1
1 1 1
1
n
n
n
n n
n
n
n n
n
u nx
u n n x
u n n x n x
n
u n n n n x x
u n n n
v n x ny
v n n x
n



 

 


 
  
    
  
    
   
   
    
 
 
2
1
1
1
1
.........................................................
1 ....3.2 1 1
! 1
! 1
n
n
n
n
n
n
n y
v n n x
n y
v n





   
 
 
  1 1 1 2 2 2 2 ....
n
n n n
n n xn
d
x y u v nc u v nc u u v uv
dx
      
     1
1! ! 1
n n
n y nc n x n y 
  
   
22 2
2
!
. . 1 1 ....
2
nn
nc x n n y 
   
     
2 21 2 2
1 2! ... 1
nn n n n
n y nc y x nc y x x       
 
.

40
Homework Problems
1. 3
.x
y x e Ans :     3 2
3 . 3 1 1 2      x x x x
ny e x ne x n n e x n n n e .
2. 2
.x
y x a Ans :    
12
log . . log . 2

 
n nx x
ny a a x n a a x    
2
1 . log

 
nx
n n a a .
3. 2
siny x x Ans : 2
sin 2 sin 1
2 2
n
n
y x x n x n x
    
       
   
 1 sin 2
2
n n x n x
 
    
 
4. 2 mx
y x e Ans :  2 1 2
. . . 2 1 . . 
   n mx n mx n mx
ny m e x n m e x n n m e
5. 3
sin 2y x x
Ans : 3 2 1
2 sin 2 . .3 2 sin 2 1.
2 2
    
       
   
n n
n
n
y x x n x x n   2
1 .3 2 sin 2 2.
2
n
n n x x n
  
    
 
   3
1 2 2 sin 2 3.
2
n
n n n x n
  
     
 
.
6.  
2
2 3 .x
y x e  Ans :      
2
2 3 .4 2 3 1 4x x x
ny e x ne x n n e     
7.  
3
3 sin3y x x  Ans :    
3 2
3 sin 3 . 3 .3 sin 3 1. . 3
2 2
n n
n
n
y x x n x n x
    
         
   
   
  
1
3
1 3 sin 3 2. . 3
2
1 2 3 sin 3 3. .
2
n
n
n n x n x
n n n x n




 
     
 
 
     
 
8. If 1
cos ,y x
 prove that    2 2
2 11 2 1 0n n nx y n xy n y     
9. If 1
sin ,y x
 prove that    2 2
2 11 2 1 0n n nx y n xy n y     
Also find  9 0y and  10 0y Ans :   2 2 2 2
9 0 1 .3 .5 .7y  and  10 0 0y  (M.U.2003)
10. If  2 2
,
m
y x a x   prove that at 0x  ,  2 2 2
2 0n na y n m y    . (M.U.1992)
11. If 1
tan ,y x
 prove that      2
2 11 2 1 1 0n n nx y n xy n n y      
Hence deduce that  0 0ny  if n is even and    0 1 !ny n  if n is odd.
12. If  
2
2 2
,y x a x   prove that      2 2 2
2 12 1 4 0n n na x y n xy n y      
13. If coslog sinlog ,y a x b x  prove that,    2 2
2 12 1 1 0n n nx y n xy n y      . (M.U. 02, 04, 05)
14. If
1
,
1
r
x
y
x
 
  
 
prove that,      2
1 11 2 1 0n n nx y r nx y n n y      
15. If  2
1 ,
n
y x  prove that,    2
2 11 2 1 0n n nx y xy n n y      (M.U.1987, 96)
16. If  1 . ,x
y x e
  
  prove that    1 11 0n n nx y n x y n y      
17. If  1
sin siny m x
 or if 1 1
sin sin ,m x y 
 prove that
     2 2 2
2 11 2 1 0n n nx y n xy m n y       (M.U.2000, 02, 04, 05)
Hence deduce that  0 0ny  if n is even and       2 2 2 2 2 2
0 ....... 3 1ny n m m m m    if n is
odd
18. If
1
sin
, 
 x
y e prove that      2 2 2
2 11 2 1 0n n nx y n xy n y      
19. If  
21
sin ,y x
 prove that    2 2
2 11 2 1 0n n nx y n xy n y      (M.U.1988, 97)

41
20. If  
1/22 1
1 sin ,y x x
  prove that      2
1 11 2 1 2 0n n nx y n xy n n y       (M.U.1995)
21. If
1
cos
,

 m x
y e prove that      2 2 2
2 11 2 1 0n n nx y n xy n m y       (M.U.1995)
22. If 1
sin log ,
n
y x
b n
    
   
   
prove that  2 2
2 12 1 2 0.n n nx y n xy n y    
23. If 1/ 1/
2 ,m m
y y x
  prove that,      2 2 2
2 11 2 1 0n n nx y n xy n m y       (M.U.2007)
24. If
1
cosh log ,x y
m
 
  
 
prove that      2 2 2
2 11 2 1 0n n nx y n xy n m y       (M.U.1993)
25. If 1
sinh ,y x
 prove that    2 2
2 11 2 1 0n n nx y n xy n y      (M.U.1987)
26. If  2
cos log 2 1 ,y x x     prove that       2 2
2 11 2 1 1 4 0n n nx y n x y n y       
27. If
log
,
x
y
x
 prove that
 
1
1 . ! 1 1 1
log 1 .....
2 3
n
n n
n
y x
x n
  
      
(M.U.1991, 96)
28. If
1
,
1
x
y
x
 
  
 
prove that  2
11y x y  and hence, prove that
      2
1 21 2 1 1 1 2 0n n nx y n x y n n y           (M.U.1983, 2004, 06)
29. If log ,n
y x x prove that  2 2
2 12 1 0x y n xy n y   
and hence, prove that    
22
2 12 2 1 0p p px y p n xy p n y       (M.U.1984, 88)
30. If  2
sinlog 2 1y x x   prove that       2 2
2 11 2 1 1 4 0n n nx y n x y n y       
31. If  cos log ,y m x show that    2 2 2
2 12 1 0n n nx y n xy m n y     
32. If 1
tan
a x
y
a x
  
   
prove that      2 2
2 12 1 1 0n n na x y n xy n n y       (M.U.1998, 2002)
33. If 1
sec ,y x
 prove that      2 2
2 11 2 3 1n nx x y n x n y 
          2
13 1 1 0n nn n xy n n y     
34. If  
2
2 2
log ,y x x a   prove that    2 2 2
2 12 1 0n n nx a y n xy n y      (M.U.1998)
35. If  
2
2
log 1y x x   
  
2 0 0n ny n y   (M.U.1997)
36. If    2
/ 2 ,y Lx M x Bx C    prove that
 
  
 
 
2
2 1
2
2 0
1 2 1
n n n
x Bx C x B
y y y
n n n
 
  
  
  
37. If
1
2
sinh
,
1
x
y
x



prove that      
22
2 11 2 3 1 0n n nx y n xy n y      
38. If cos ,t
x e y mt  prove that    2 2 2
2 12 1 0n n nx y n xy m n y    
39. If
1
cos ; log ,x y
m
   prove that      2 2 2
2 11 2 1 0n n nx y n xy n m y       .

42
Extra Solved Examples
1. If  1 ,
n
y x  prove that 31 2
.......
1! 2! 3! !
nny yy y
y x
n
     
Soln
: We have  1
n
y x 
    
        
1 2
1 2
3 4
3 4
1 1 1
1 2 1 1 2 3 1
n n
n n
y n x y n n x
y n n n x y n n n n x
 
 
     
        
………………………………………. ……………………………………………
        
   
 
 
0
1 2
1 2 ....... 1 1 ! 1
1
. . . 1 1 1
1! 2!
n n
n
n n n
y n n n n n x n x
n nn
I h s x x x

 
          

      
  
   
3 01 2 !
1 ..... 1
3! !
nn n n n
x x
n
 
    
 1 1
n n
x x    (By Binomial Theorem)
Hence, the result
2. If tan ,y x prove that        2 2 4 40 0 0 ... sin / 2    n n
n n ny C y C y n
Soln
: We have cos siny x x
Now applying Leibnitz’s theorem to the left hand side
     1 1 2 2cos sin cosn n
n n ny x C y x C y x    
   3 3 4 4sin cos ... sin
2
n n
n n
n
C y x C y x x

 
 
     
 
Now put 0x  ,      2 2 4 40 0 0 ... sin
2
n n
n n n
n
y C y C y

      .
3. If  2
log 1 ,y x x    2
if e 1y
or x x  
prove that  2 0 0ny  and      
22 2 2
2 1 0 1 .1 .3 .5 .... 2 1
n
ny n    .
Soln
: Since  2
log 1y x x   …………………..(1)
1 2 2 2
1 1
1
1 1 1
x
y
x x x x
 
   
    
……………….(2)
Differentiating again,
2
2 12
1. . 0
1
  

x
x y y
x
 2
2 11 . 0x y xy    ……………………(3)
Applying Leibnitz’s Theorem
   
 
 2
2 1
1
1 . 2 . 2 .
2!
n n n
n n
x y n x y y 

    1. . 1 0n nx y n y  
   2 2
2 11 2 1 0n n nx y n xy n y       ………………….(4)
Putting 0x  in (1),(2),(3) we get
   0 log 1 0,y    1 0 1,y   2 0 0y 
Now from (4), we get,

43
   2
2 0 0n ny n y   ………………………(5)
Putting  1,2,3,4,...... 5n in
       
       
       
2 2 2
3 1 4 2
2 2 2 2
5 3 6 4
2 2 2 2 2
7 3 8 6
0 1 . 0 1 ., 0 2 . 0 0
0 3 . 0 3 .1 , 0 4 . 0 0
0 5 . 0 5 .3 .1 , 0 6 . 0 0
y y y y
y y y y
y y y y
      
     
      
Hence by generalization
 2 0 0ny  and      
22 2 2
2 1 0 1 1 .3 .5 ..... 2 1
n
ny n
   
 
.
4. If  
21
siny x
 , prove that
 0 0ny  if n odd and
   
22 2 2
0 2. 2 . 4 .6 ........ 2ny n  if 2
2n and n is even.
Soln
: We have  
21
siny x
 ……..(1)
 1
1 2
1
2 sin .
1
y x
x

 

…………(2)
2 1
11 . 2sinx y x
  
2
2 1 2 2
2
1 . .
1 1
x
x y y
x x
   
 
 2
2 11 . 2x y x y    …………..(3)
By Leibnitz’s theorem,
   
 
 2
2 1
1
1 2 2
2!
n n n
n n
x y n x y y 

      1 1 0n nx y n y    
   2 2
2 11 2 1 0n n nx y n xy n y       …………(4)
Putting    2
20, 0 0n nx y n y  …………..(5)
Putting 0,x  in (1), (2), and (3) we get,
         1 20 0 , 0 0 , 0 2,y y y  
Putting 1,3,5,............n  in (5), we get,
       2 2
3 1 5 30 1 . 0 0, 0 3 . 0 0,y y y y    
     2
7 50 5 . 0 0, 0 0ny y y   if n is odd
Putting 2,4,6,.............n  in (5), we get,
       2 2 2 2 2
4 2 6 40 2 . 0 2 .2; 0 4 . 0 4 . 2 . 2y y y y   
   
22 2 2
0 2.2 .4 .6 ....... 2ny n  if n is even and 2.n 
5. If  
21
sin ,y x
 prove that at    2
20, 0 0 .n nx y n y 
If, further,  
21 2
0 1 2sin ... .....n
ny x a a x a x a x
     
prove that    2
21 2 .n nn n a n a  
Soln
: We have proved above that,
   2
2 0 0n ny n y 
Now consider,

44
 
0 1
1
1 1 2
2
2 2
........... ......
2 ....... ......
2 ..... 1 .......
n
n
n
n
n
n
y a a x a x
y a a x n a x
y a n n a x


    
     
     
…….. ……. …….. ……
  1 2 ...........2. 1. a +.........n ny n n n  
! ........nn a 
 0 ! .n ny n a
Similarly,     22 0 2 ! nny n a   
But    2
2 0 0n ny n y  (proved above in (5))
 
  
2
2
2
2
2 ! !
1 2 .
n n
n n
n a n n a
n n a n a


  
   
6. If
1
tan
0 1 ...... ......,

     m x n
ny e a a x a x
prove that at 0,x         2 10 0 1 0 0.n n ny my n n y    
Hence, or otherwise deduce that    1 11 1 .n n nn a n a ma    
Soln
: Proceeding as in Ex. 11 page (5.28), we can prove that,
     2
2 11 2 1 1 0.n n nx y n x m y n n y         
Putting 0,x  we get
       2 10 0 1 0 0n n ny m y n n y     …………(1)
Now if,
 
2
0 1 2
1
1 1 2
1
2 2
..... .....
2 ...... .......
2 ...... 1 .......
n
n
n
n
n
n
y a a x a x a x
y a a x na x
y a n n a x


     
    
    
………… ……… ……… ……….. ………….
! ....n ny n a  as above.
Putting 0,x  we get,
 0 !n ny n a ……………..(2)
Changing n to 1n  in (1),
     1 10 0 1 . 0n n ny my n n y     ………….(3)
Putting 1n n  and 1n  in (2) we get,
       1 1 1 10 1 ! , 0 1 !n n n ny n a y n a      
Putting these values in (3)
   
   
   
1 1
1 1
1 1
1 ! . ! 1 ! 0
1 1 0
1 1
n n n
n n n
n n n
n a m n a n n a
n a m a n a
n a n a ma
 
 
 
    
     
    
7. If  log ,
n
n
n n
d
l x x
dx
 prove that  1 1 !n nl nl n  
Hence show that
1 1 1
! log 1 ........
2 3
nl n x
n
 
       

45
Soln
: We have
   
1
1
log . log
n n
n n
n n n
d d d
l x x x x
dx dx dx


 
 
 
1
1
1
1 1
1 1
1 1
1
1
log .
.log
1 !
n
n n
n
n n
n n
n n
n
d
nx x x
dx x
d d
n x x x
dx dx
n l n



 
 
 

 
   
   
  
Dividing both sides by !n we get,
  1
1 1
! 1 !
n
n
l
l
n n n
 

Putting 2,3,4,...... ,n n
 
2
1
3
2
2
3
1
1 1
.
2! 1! 2
1 1
.
3! 2! 3
1 1
.
4! 3! 4
..... .... .... ....
..... .... .... ....
1 1
.
! 1 !
n
n
l
l
l
l
l
l
l
l
n n n

 
 
 


 

Adding all these equalities, we get,
1
1 1 1 1
............
! 2 3 4
nl
l
n n
     
Now  1 log 1 log
d
l x x x
dx
  
1 1 1
! log 1 ......
2 3
nl n x
n
 
        
.
8. If  1
log ,n n
nU D x x
 prove that   11n nU n U   and hence deduct that
 1 !
n
n
U
x

 .
Soln
: We have
   
1
1 1
1
log . log
n n
n n
n n n
d d d
U x x x x
dx dx dx

 

 
 
   
   
 
1
2 1
1
1
1 1 2
1
1 1
1 1 2
1 1
1
1 .log .
1 .log
1 .log
n
n n
n
n
n n
n
n n
n n
n n
d
n x x x
dx x
d
n x x x
dx
d d
n x x x
dx dx

 


  

 
  
 
 
    
    
    
  11 nn U   (Second term is zero)
Applying the result repeatedly,
   21 2n nU n n U   

46
   
   
 
3
1
1
1 2 3
1 2 3 ...........3. 2. 1.
1 !.
nn n n U
n n n U
n U
   
   
 
But    0
1
1
log log
d d
U x x x
dx dx x
  
 
1
1 !nU n
x
   .
9. If  2
1 ,
n
y x  prove that    2
2 11 2 1 0.n n nx y xy n n y     
Hence deduct that if  2
1 ,
n
n
n n
d
l x
dx
  then    2
1 1 .n
n
x
dld
x n n l
dx d
 
    
 
Soln
: We have  2
1
n
y x 
 
12
1 1 .2
n
y n x x

  
   2 2
11 1 .2 2
n
x y n x x n x y    
 2
2 1 11 2 2 2x y x y n x y n y   
   2
2 11 2 1 y 2 y = 0x y n x n    
Now by Leibnitz’s Theorem
   
 2
2 1
1
1 2 .2
2!
n n n
n n
x y n x y y 

  
  12 1 2 0n n nn xy ny n y    
   2
2 11 2 1 0n n nx y xy n n y       ………………..(1)
We first note that
   2
1
n n
n
n nn n
d d
l x y y
dx dx
  
Now  2
1 ndld
x
dx dx
 
 
 
   
  
 
2
2
1
2
2 1
1
1
1 2
n
n
n n
d d
x y
dx dx
d
x y
dx
x y x y

 
 
  
 
 
  
 1 nn n y   from (1) .
10. If u is a function of x and ,ax
y e u Prove that   .
nn ax
D y e D a u 
Soln
: Let ax
v e then 1
ax
v ae
2 3
2 3, ,......ax ax n ax
nv a e v a e v a e  
By Leibnitz’s theorem,
  0 1 1 1
n n n
n nD y D uv C u v nC u v  
2 2 2 ... n
n n nnC u v C uv  
Treating D as an operator, we write .n
nD u u

47
1 2
0 1 1 2 2. . .n n n n n n n
D y C D u v C D u v C D u v 
   
3
3 3. ..... . .n n n
n nC D u v C u v
  
1 2 2
0 1 2. . .n n ax n n ax n n ax
C D u e C D u ae C D u a e 
  
3 3
3 . .... .n n ax n n ax
nC D u a e C u a e
  
1 2 2 3 3
0 1 2 3
1 2 2 3 3
0 1 2 3
. . . . .... .
. ....
ax n n n n n n n n n n
n
ax n n n n n n n n n n
n
e C D u C D u a C D u a C D u a C u a
e C D C D a C D a C D a C a u
  
  
       
       
  . .
nax
e D a u 
11. If  
2
2
log 1 ,y x x   
  
prove that    2 2
2 11 2 1 0n n nx y n xy n y     
Hence, deduce that    2
2 0 0n ny n y  
Soln
: We have  
2
2
log 1y x x   
  
…………….(1)
Differentiating it w.r.t.x, we get,
 2
1 2 2
1
2 log 1 . 1
1 1
x
y x x
x x x
            
 2
2
1
2 log 1 .
1
x x
x
   
   
 2 2
11 y 2.log 1x x x    ……………….(2)
2
2 12 2 2
1
1 y y 2. 1
1 1 1
x x
x
x x x x
 
    
    
2
2
1 x


 2
2 11 2x y xy    …………………..(3)
Applying Leibniz’s theorem, we get,
   
 
 2
2 1
1
1 . 2 2
2!
n n n
n n
x y n x y y 

  
 1 . 1 0n nxy n y  
   2 2
2 11 2 1 0n n nx y n xy n y       …………….(4)
Putting 0x  in (4), we get    2
2 0 0n ny n y   .
12. Using Leibnitz theorem for 2n
x prove that
       
 
2 2 22 22
22 2 2 2 2
1 1 2 2 !
1 ....
1! 1 .2 1 .2 .3 !
n n n n n nn
n
  
    
Soln
: We obtain nth
derivative of 2n
y x in two different ways.
By applying the formula (2), page (5.2),
     2
2 2 1 2 2 ...... 2 1 n n
ny n n n n n x 
    
      
 
 
2 2 1 2 2 .... 1 . ...3.2.1
1 ....3.2.1
2 !
!
n
n
n n n n n
x
n n
n
x
n
  




48
By applying Leibnitz’s rule to .n n
y x x we have
     1
. .n n n n n n
ny D x x nD x D x
 
 
   2 21
2!
n n nn n
D x D x

  
   3 31 2
....
3!
n n nn n n
D x D x 
 
 
 1!
! .
1!
n nn x
n x n n x 
 
 
  
  
   
2 2
3 3
1 !
1
2! 2!
1 2 !
1 2
3! 3!
...........
n
n
n n n
x n n x
n n n n
x n n n x


  
  
 
   
   
 

     
2 2 22 22
2 2 2 2 2 2
1 1 2
! 1 ........
1 1 .2 1 .2 .3
n n n n n nn
n x
   
     
  
Equating the two results, we get
     
2 2 22 22
2 2 2 2 2 2
1 1 2
! 1 ........
1 1 .2 1 .2 .3
n n n n nn
n
   
     
  
 2 !
!
n
n

       
 
2 2 22 22
22 2 2 2 2 2
1 1 2 2 !
1 ........
1 1 .2 1 .2 .3 !
n n n n n nn
n
  
      .

Successive differentiation

Recommandé

Recommandé

Contenu connexe

Tendances

Tendances (20)

Successive differentiation