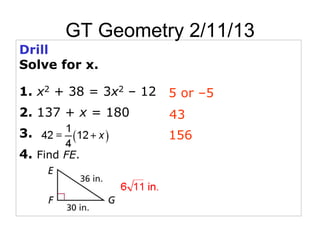
GT Geometry 2/11/13
Drill
Solve for x.
1. x2 + 38 = 3x2 – 12 5 or –5
2. 137 + x = 180 43
3. 156
4. Find FE.

Objectives
Use properties of kites to solve
problems.
Use properties of trapezoids to solve
problems.

Vocabulary
kite
trapezoid
base of a trapezoid
leg of a trapezoid
base angle of a trapezoid
isosceles trapezoid
midsegment of a trapezoid

A kite is a quadrilateral with exactly two pairs of
congruent consecutive sides.

Example 1: Problem-Solving Application
Lucy is framing a kite with
wooden dowels. She uses two
dowels that measure 18 cm,
one dowel that measures 30
cm, and two dowels that
measure 27 cm. To complete
the kite, she needs a dowel to
place along . She has a dowel
that is 36 cm long. About how
much wood will she have left
after cutting the last dowel?

Example 1 Continued
3 Solve
N bisects JM.
Pythagorean Thm.
Pythagorean Thm.

Example 1 Continued
Lucy needs to cut the dowel to be 32.4 cm long.
The amount of wood that will remain after the
cut is,
36 – 32.4 3.6 cm
Lucy will have 3.6 cm of wood left over after the
cut.

Example 1 Continued
4 Look Back
To estimate the length of the diagonal, change the
side length into decimals and round. , and
. The length of the diagonal is
approximately 10 + 22 = 32. So the wood
remaining is approximately 36 – 32 = 4. So 3.6 is a
reasonable answer.

Check It Out! Example 1
What if...? Daryl is going to make
a kite by doubling all the measures
in the kite. What is the total
amount of binding needed to cover
the edges of his kite? How many
packages of binding must Daryl
buy?

Check It Out! Example 1 Continued
3 Solve
Pyth. Thm.
Pyth. Thm.
perimeter of PQRS =

Check It Out! Example 1 Continued
Daryl needs approximately 191.3 inches of binding.
One package of binding contains 2 yards, or 72 inches.
packages of binding
In order to have enough, Daryl must buy 3 packages
of binding.

Check It Out! Example 1 Continued
4 Look Back
To estimate the perimeter, change the side lengths
into decimals and round.
, and . The perimeter of the
kite is approximately
2(54) + 2 (41) = 190. So 191.3 is a reasonable
answer.

Example 2A: Using Properties of Kites
In kite ABCD, m DAB = 54°, and
m CDF = 52°. Find m BCD.
Kite  cons. sides
∆BCD is isos. 2 sides isos. ∆
CBF CDF isos. ∆ base s
m CBF = m CDF Def. of s
m BCD + m CBF + m CDF = 180° Polygon Sum Thm.

Example 2A Continued
m BCD + m CBF + m CDF = 180°
Substitute m CDF
m BCD + m CDF + m CDF = 180°
for m CBF.
Substitute 52 for
m BCD + 52° + 52° = 180°
m CDF.
m BCD = 76° Subtract 104
from both sides.

A trapezoid is a quadrilateral with exactly one pair of
parallel sides. Each of the parallel sides is called a
base. The nonparallel sides are called legs. Base
angles of a trapezoid are two consecutive angles
whose common side is a base.

If the legs of a trapezoid are congruent, the trapezoid
is an isosceles trapezoid. The following theorems
state the properties of an isosceles trapezoid.

Example 3A: Using Properties of Isosceles
Trapezoids
Find m A.
m C + m B = 180° Same-Side Int. s Thm.
100 + m B = 180 Substitute 100 for m C.
m B = 80° Subtract 100 from both sides.
A B Isos. trap. s base
m A=m B Def. of s
m A = 80° Substitute 80 for m B

Example 3B: Using Properties of Isosceles
Trapezoids
KB = 21.9 and MF = 32.7.
Find FB.
Isos.  trap. s base
KJ = FM Def. of segs.
KJ = 32.7 Substitute 32.7 for FM.
KB + BJ = KJ Seg. Add. Post.
21.9 + BJ = 32.7 Substitute 21.9 for KB and 32.7 for KJ.
BJ = 10.8 Subtract 21.9 from both sides.

The midsegment of a trapezoid is the segment
whose endpoints are the midpoints of the legs. In
Lesson 5-1, you studied the Triangle Midsegment
Theorem. The Trapezoid Midsegment Theorem is
similar to it.

Example 5: Finding Lengths Using Midsegments
Find EF.
Trap. Midsegment Thm.
Substitute the given values.
EF = 10.75 Solve.