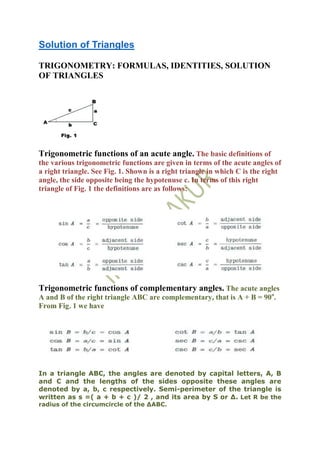
1. Solution of Triangles
TRIGONOMETRY: FORMULAS, IDENTITIES, SOLUTION
OF TRIANGLES
Trigonometric functions of an acute angle. The basic definitions of
the various trigonometric functions are given in terms of the acute angles of
a right triangle. See Fig. 1. Shown is a right triangle in which C is the right
angle, the side opposite being the hypotenuse c. In terms of this right
triangle of Fig. 1 the definitions are as follows:
Trigonometric functions of complementary angles. The acute angles
A and B of the right triangle ABC are complementary, that is A + B = 90o.
From Fig. 1 we have
In a triangle ABC, the angles are denoted by capital letters, A, B
and C and the lengths of the sides opposite these angles are
denoted by a, b, c respectively. Semi-perimeter of the triangle is
written as s =( a + b + c )/ 2 , and its area by S or Δ. Let R be the
radius of the circumcircle of the ΔABC.

2. Basic Formulae
If the three angles A, B, C are given, we can only find the ratios of
the sides a, b, c by using the sine rule (since there are infinite
similar triangles possible).
In trigonometry, the law of sines (also known as the sine law, sine
formula, or sine rule) is an equation relating the lengths of the sides
of an arbitrary triangle to the sines of its angles. According to the
law,
where a, b, and c are the lengths of the sides of a triangle, and A, B,
and C are the opposite angles (see the figure to the right). Sometimes
the law is stated using the reciprocal of this equation:
The law of sines can be used to compute the remaining sides of a
triangle when two angles and a side are known—a technique known
as triangulation. It can also be used when two sides and one of the
non-enclosed angles are known. In some such cases, the formula gives
two possible values for the enclosed angle, leading to an ambiguous
case.
The law of sines is one of two trigonometric equations commonly
applied to find lengths and angles in a general triangle, the other
being the law of cosines
(i) Sine rule
A
c b
B C
D a

3. Case i When △ABC is an acute angled triangle:
AD is per. on BC , in △ABD sinB = AD/AB ⇨ csinB = AD....(i)
In △ ACD , AD = bsinC......(ii), from (i) & (ii) b/sinB = c/sinC
Similarly a/sinA = c/sinC
Case (ii) When △ABC is an obtuse angled triangle
A
C b
D B C
a
AD is per. on BC , in △ABD sin<ABD = AD/AB=sin(1800-B)=sinB ⇨
c sinB = AD....(i)
In △ ACD , AD = bsinC......(ii), from (i) & (ii) b/sinB = c/sinC
Similarly a/sinA = c/sinC
Case(iii)When △ABC is rightangled triangle
SinC = 1, SinA = BC/AB = a/c & sinB = AC/AB = b/c
∴ sin A/a = sin B/b = sinC/c = 1/2R = 2Δ/abc. [R=abc/4△]
.radius of circumcircle or R=a/2sinA=b/2sinB=c/2sinC,
△=(1/2)bcsinA and radius of incircle is r=△/s or r =(s-a)tan(A/2)
r =(s-b)tan(B/2), r=(s-c)tan(C/2) and
r=4Rsin(A/2)sinB/2sinC/2
Example 1. In △ABC, if a=2, b=3 and sinA =2/3 , find <B.
[hint: by using law of sine , we get = , <B=п/2]
In △ABC, if the angles are in the ratio of 1:2:3. Prove that the corresponding
Sides are in the ratio of 1:√3:2.

4. [hint: angles are Ѳ+2Ѳ+3Ѳ=180⇨ 300, 600 ,900 ⇨ = = ]
(ii) Cosine rule
CASE(i) cosB = BD/c ⇨ BD = c cosB & CD = bcosC
By Pythagoras thm. AC2 = AD2+CD2 = AD2+(BC – BD)2
AC2 =BC2 +(AD2+BD2) – 2. BC.BD= BC2 + AB2– 2. BC.BD
⇨ b2 = c2+a2 – 2cacosB
Case(ii) cos(1800-B) = BD/c ⇨ BD = -c cosB & CD = bcosC AC2 =
AD2+CD2 = AD2+(BC +BD)2
AC2 =BC2 +(AD2+BD2) + 2. BC.BD= BC2 + AB2+ 2. BC.BD⇨ b2 =
c2+a2 – 2cacosB
CASE(iii) ⇨ b2 = c2+a2 ⇨ b2 = c2+a2 – 2cacosB
∴cos A =( b2 + c2 - a2 )/2abc cosB =( a2 + c2 - b2 ) /2ac ,
cosC = (a2 + b2 - c2)/2ab .
(iii) Trigonometric ratios of half-angles:
sin A/2 = √[(s-b) (s-c)]/bc, cos B/2 = √[s(s - b)]/ca,
tan C/2 = √ [(s - a) (s - b)]/[s(s - c)] .
(iv) Projection rule:
In case (i) cosB=BD/AB ⇨ BD=cCosB & cosC=CD/AC ⇨ CD=bcosC
∴ a = BC=BD+CD = cCosB+ bcosC
In case (ii) cos(1800-B)= BD/AB ⇨ BD= - cCosB
∴ a = BC= CD - BD = cCosB+ bcosC
a = b cosC + c cosB, b = c cosA + a cosC, c = a cosB + b cosA.
(v) Area of a triangle
In case (i) sinB= AD/AB ⇨ AD=csinB ∴ △ = ½. BC.AD=1/2.acsinB
In case(ii) sin(1800-B)= AD/AB ⇨ AD= - csinB
Δ = 1/2 bc sin A = 1/2 ca sinB = 1/2 ab sin C = √[s(s - a) (s - b)
(s - c)] = abc/4R = rs.

5. (vi) Napier’s analogy
tan (B – C)/2 =( b – c)/(b +c) cot A/2 , tan (C – A)/2 = (c –a)/(
c + a) cot B/2 , tan (A – B)/2 = (a – b) /(a + b) cot c/2.
**(vii) m-n theorem
If D be the point on the side BC of a triangle ABC which divide the
side BC in the ratio m : n, then with respect to mentioned figure,
we have:
(i) (m + n) cot θ = m cot α – n cot ß.
(ii)(m + n) cot θ = n cot B – m cot C.
**(viii) Apollonius theorem
In a triangle ABC, AD is median through A, then AB2 + AC2 =
2(AD2+BD2).
Process of Solution of Triangles
The three sides a, b, c and the three angles A, B, C are called the
elements of the triangle ABC. When any three of these six
elements (except all the three angles) of a triangle are given, the
triangle is known completely; that is the other three elements can
be expressed in terms of the given elements and can be
evaluated. This process is called the solution of triangles.
(i) If the three sides a, b, c are given, angle A is obtained from
tan A/2 = √[(s - b) (s - c)] / [s(s - a)] or cos A = (b2 + c2 - a2 )/
2bc . B and C can be obtained in the similar way.
(ii) If two sides b and c and the included angle A are given, then
tan (B – c)/2 = (b – c)/ (b + c) cot A/2 gives (B – C)/2. Also
( B+ C)/2 = 90o - A/2, so that B and C can be evaluated. The third
side is given by a = b sin A/sin B or a2 = b2 + c2 – 2bc cosA.

6. *(iii) If two sides b and c and the angle B (opposite to side b) are
given, then sin C = c/b sinB, A = 180o – (B + C) and b = b sin
A/sinB give the remaining elements. If b < c sin B, there is no
triangle possible (Fig. 1) If b = c sinB and B is an acute angle,
then only one triangle is possible (Fig. 2) If c sinB < b < c and B is
an acute angle, then there are two values of angle C (Fig. 3). If c
< b and B is an acute angle, then there is only one triangle (Fig.
4).
This is, sometimes, called an ambiguous case.
In trigonometry, the law of cosines (also known as the cosine formula
or cosine rule) relates the lengths of the sides of a plane triangle to
the cosine of one of its angles. Using notation as in Fig. 1, the law of
cosines says
where γ denotes the angle contained between sides of lengths a and b
and opposite the side of length c.
The law of cosines generalizes the Pythagorean theorem, which holds
only for right triangles: if the angle γ is a right angle (of measure 90°
or π/2 radians), then cos(γ) = 0, and thus the law of cosines reduces to

7. The law of cosines is useful for computing the third side of a triangle
when two sides and their enclosed angle are known, and in
computing the angles of a triangle if all three sides are known.
By changing which sides of the triangle play the roles of a, b, and c in
the original formula, one discovers that the following two formulas
also state the law of cosines:
In trigonometry, the law of tangents is a statement about the relationship
between the tangents of two angles of a triangle and the lengths of the
opposite sides.
In Figure , a, b, and c are the lengths of the three sides of the triangle, and
α, β, and γ are the angles opposite those three respective sides. The law of
tangents states that
The law of tangents, although not as commonly known as the law of
sines or the law of cosines, is equivalent to the law of sines, and can be
used in any case where two sides and a non-included angle, or two
angles and a side, are known.
Proof
To prove the law of tangents we can start with the law of sines:
Let
so that
It follows that

8. Using the trigonometric identity, the factor formula for sines
specifically
we get
As an alternative to using the identity for the sum or
difference of two sines, one may cite the trigonometric
identity
SOME QUESTIONS BANK
Q.1 In △ABC , prove that (i) =
(ii) a sin(B-C) + b sin(C-A) + c sin(A-B) = 0
[hint : put a = ksinA, b= ksin B & c= sinC by law of sine
On the R.H.S of (i) k2(sin2B – sin2C) / k2 sin2A ⇨
= = =
L.H.S of (ii) k[ sinA.sin(B-C)+ sinB.sin(C-A)+ sinC.sin(A-B)]
K [sin(B+C). sin(B-C)+ sin(C+A).sin(C-A)+ sin(A+B).sin(A-B)]
K[sin2B-sin2C + sin2C-sin2A+ sin2A-sin2B] = 0
Q.2 In △ABC, prove that:

9. (i) = cos(A/2) (ii) a = (b+c) sin(A/2)
(iii) (b-c) cot(A/2)+ (c-a) cot(B/2) + (a-b) cot(C/2) =0
[HINT: put the values of a,b,c by law of sines and use A+B+C= on the
R.H.S. of (i) & (ii) , but in (iii) take L.H.S. , we get K[2
cot(A/2) + 2 cot(B/2)+ 2 cot(C/2)] =
k[2cos(A/2) + 2cos(B/2) + 2cos(C/2) ]
Q.3 In a △ABC, if a= 2, b= 3 and sinA = 2/3 , find angle B.
[hint: use a/sinA = b/ sinB = c/sinC ⇨ 2/(2/3) = 3/sinB ⇨ sinB = 1 ⇨ B=900]
Q.4 In △ ABC, if acosA = bcosB , show that the triangle is either isosceles
or right angled.
[hint: ksinAcosA = k sinBcosB ⇨ sin2A = sin2B ⇨ 2A=2B or 2A = п-2B
A=B or A+B=п/2]
Q.5 In any △ ABC , P.T.
(i) a(bcosC – ccosB) = b2-c2 (ii) (a-b)2 cos2(C/2) + (a+b)2 sin2(C/2) =c2
[hint: L.H.S ab{ (a2 + b2 - c2)/2ab} –ac {( a2 + c2 - b2 ) /2ac}= b2-c2
(ii) L.H.S a2 [cos2(C/2) + sin2(C/2)]+ b2 [cos2(C/2) + sin2(C/2)]-
2ab[cos2(C/2) - sin2(C/2)]= a2+b2 – 2abcosC = c2 ]
Q.6 In any △ ABC , P.T.
(i) = (ii) 2(asin2(C/2)+csin2(A/2)) = a-b+c
(iii) 2(acos2(C/2)+ccos2(B/2)) = a+b+c
(iv) (b+c)cosA+(c+a)cosB+(a+b)cosC=a+b+c
[hint: (i) R.H.S = = b/c
(ii) [a(1-cosC)+ c(1-cosA)]= a-b+c
(iii) same as (ii), (iv) + +

10. =a+b+c by projection formulas ]
Q.7 In △ ABC, angle C= 600 , then p.t. + =
[hint: cosC = ½ ⇨ (a2 + b2 - c2)/2ab =1/2 ⇨ a2 + b2 – ab= c2
Now + = if = if a2 + b2 – ab= c2 ]
Q.8 Find the area of a △ABC in which angle A = 600 ,b=4 cm and
c= .
[△ = ½ . bc sinA = ½ 4 . sin60 = 3 sq. Cm.]
Q. 9 In △ ABC, if a=13 , b=14 and c=15, find the following:
(i) △ (ii) sin(A/2) (iii) cos(A/2) (iii) tan(A/2) (iv) sinA (v) cosA (vi) tanA
[HINT: s= (a+b+c)/2 = 21 , △ = =84
Sin(A/2) = =√(1/5), cos(A/2) = =2/√5
Tan(A/2) = =1/2 , △ = ½.bc sinA ⇨ sinA = 4/5
,cosA=3/5 , tan A = 4/3]
Q.10 In △ ABC, bcosB+ ccosC = acos(B-C).
[HINT: L.H.S k(sinBcosB + sinC cosC) =k/2 (sin2B+sin2C) =k/2
(2sin(B+C)cos(B-C)) = ksin(1800-A)cos(B-C).]
Q. 11 a( sinB-sinC) + b (sinC-sinA) + c( sinA-sinB) = 0. [ use law of sine]
Q.12 In △ ABC, p.t. =
[HINT: L.H.S = , use
)= cos(A+B).cos(A-B)]
Q. 13 In △ ABC, P.T. (i) = (ii) = [use law
of sin on L.H.S for (i) & (ii) ]

11. (iii) a(cosC-cosB) = 2(b-c)cos2(A/2) [put 2cos2(A/2)= 1+cosA)on
R.H.S & use projection formulas] (iv) asinA - bsinB = csin(A-B)
[put a=ksinA & b=ksinB on L.H.S &use formula of sin2A-sin2B
=sin(A+B).sin(A-B)] (v) a(bcosC – ccosB) = b2-c2 [ use cosC =
(a2+b2-c2)/2ab]
Q.14 In △ ABC, (i) if (b+c)/12=(c+a)/13=(a+b)/15 then p.t.
cosA/2=cosB/7=cosC/11 [ let (b+c)/12=(c+a)/13=(a+b)/15=k ⇨ a+b+c=20k
2 2
⇨ a= 8k, b=7k & c=5k , we get cosA = 1/7, cosB= ½ & cosC = (a +b -
c2)/2ab = 11/14 ,cosA: cosB: cosC= 1/7:1/2:11/14 = 2:7:11]
(ii) p.t. = [use cos A = on L.H.S]
(iii) a=18,b=24 & c=30,find cosA , cosB , cosC [use cos A =
,ans. are 4/5, 3/5 0 resp.]
(iv) 2(bccosA+cacosB+abcosC) = a2+b2+c2 [use cos A = ]
(v) p.t. sin2A+ sin2B+ sin2C=0
[HINT: 2sinAcosA+ 2sinBcosB+ 2sinCcosC
Then put sinA , sinB ,sinC as ak,bk,ck resp.& cos A = ]
(vi) p.t + + = [use cos A = on L.H.S]
(vi) a=4,b=6 &c=8,show that 6cosC=4+3cosB
(vii) if <B=600 , P.T (a+b+c)(a-b+c)=3ac
(viii)p.t. (b2-c2)cotA+(c2-a2)cotB+(a2-b2)cotC=0
[hint: (b2-c2)cosA/sinA+(c2-a2)cosB/sinB+(a2-b2)cosC/sinC , use
sinA=ak & cos A = ]
(ix) if cosC= sinA/(2sinB), p.t. triangle is an isosceles.

12. [put sinA=ak ,sinB=bk⇨ 2cosAsinC=sinB ⇨ 2 )kc=kb ]
(x) p.t (a-b)2cos2(C/2)+(a+b)2sin2(C/2)=c2
[hint: a2(cos2(C/2)+sin2(C/2)) + b2 (cos2(C/2)+sin2(C/2))-
2ab(cos2(C/2)-sin2(C/2)) =a2+b2-2abcosC=c2 ]
(xi) p.t a2=(b+c)2-4bccos2(A/2)
(xii) p.t. (c2+b2-a2)tanA=(a2+c2-b2)tanB=(a2+b2-c2)tanC
[hint: (c2+b2-a2)sinA/cosA=(a2+c2-b2)sinB/cosB= (a2+b2-
c2)sinC/cosC law of sin & cosine formulas]
We have studied that a triangle has six parts or six elements viz
three sides and three angles. From geometry, we know that when
any three elements are given of which necessarily a side is given,
the triangle is completely determined i.e, remaining three elements
can be determined. The process of determining the unknown
elements knowing the known elements is known as the solution of
a triangle. In practice, there are four different cases for which the
solution is discussed as under.
Case I
When all three sides are given.
To solve a triangle given the three sides a, b, c.
To determine angles A, B and C.
The angles A, B and C are determined by using the following
relations:

13. Example:
The sides of a triangle are 20, 30, and 21. Find the greatest angle.
Suggested answer:
The greatest angle is opposite to the side whose length is 30.
Let a = 20, b = 30, c = 21.
We have to find angle B.
Case 2:
When two sides and the angle included between these are given.
Example:
If b = 251, C = 147, A = 47o, find the remaining angles.
(Use Napier's rule)
Suggested answer:

14. = 0.268 x 2.2998
Case 3:
To solve a triangle having given two angles and a side.
Let the given parts be denoted by B, C, a, then the third angle A can
be found from the relation.
Similarly, c can be found from the equation.
log c = log a + log sin C - log sin A
Example:

15. Solve the triangle ABC, given a =18, A = 25o, B = 108o.
Suggested answer:
C = 180o - (25 + 108o) = 47o
Taking log on both sides, we get
log b = log a + log (sin B) - log (sin A)
= log 18 + log (sin 180o) - log (25o)
= log 18 + log (sin 72o) - log (25o)
= log 18 + log (0.9511) - log (0.4226)
(from Trigonometric table)
= 1.2553 - 1 + 0.9782 + 1 - 0.6295
log b = 1.6076
Taking antilog, we get b = 40.52.
Similarly using
log c = log a + log (sin C) - log (sin A),
we can evaluate the value of C as 31.23o.
Case 4:
When two sides b, c and an angle B opposite to one of the given
sides are given.

16. log sin C = log c + log B - log b which determines C.
The equation (i) in general leads to two solutions say C1 and C2 (say
such that C1 + C2 = 180), suppose C1 is acute.
Corresponding to C1 and C2, we have two values a1, a2 (say of the
remaining side a). They are given by
From equation (i), if an acute angle C1 is a solution of (i) then
180o - C1 is also a solution and there is an ambiguity. However if
From geometry, C must be acute. For if C is 90o or more than B
then C must be greater than 90o. This is impossible, since a triangle
can have only one right angle or more in size.
If b < c, there may be two triangles having the given elements b, c
and B. One having an acute angle C1 and second having an angle
Fig (a) Fig (b)

17. Fig (c)
Figures (a) and (b) show its two triangles.
Figure (c) shows two triangles superimposed and suggests a
geometric method of drawing the two triangles.
From figure (c), it is evident that if b < c sinB, there is no triangle.
The following rules may be used to solve a triangle with given b, c
and B.
Step 1:
Use (i) to find log (sin C).
Step 2:
If log (sin C) comes out as positive, sin C would have to be greater
than 1 and there is no solution.
Step 3:
If log (sin C) comes out as negative or zero, find the corresponding
acute angle C.
Then, find A = 180o - (B + C) and finally get 'a' by using the law of
sines (this completes the solution of ).
Step 4:
If bo - C instead of C.
Example:
If in a DABC, a = 97, b = 119, A = 50o, find B and c given that:

18. log (sin (70o, 1')) 9.9730318 .
Suggested answer:
B = 70o0'57" or (180o - 70o0'57") i.e., 109o59'03"
Since a < b, both the values of B is admissible and then case is
ambiguous.