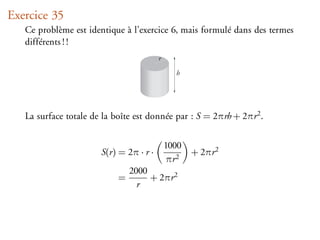
1. Exercice 35
Ce problème est identique à l’exercice 6, mais formulé dans des termes
différents ! !
r
h
La surface totale de la boîte est donnée par : S = 2πrh + 2πr 2 .
1000
S(r) = 2π · r · + 2πr 2
πr 2
2000
= + 2πr 2
r

2. Exercice 35 (suite..)
Le volume est maximum lorsque :
−2000
S (r) = + 4πr = 0
r2
⇒ 4πr 3 = 2000
2000
3
r= 5, 419 cm.
4π
1000
h= 10, 84 cm.
π · 5, 4192
Il s’agit bien d’un minimum, car lorsque r = 5, 419, S (5, 419) > 0
Ainsi :
Rayon : 5,419 cm et Hauteur : 10,84 cm.